Conpackt, A Contouring Package for Triangular Meshes

David J. Kennison
NCAR, P.O. Box 3000, Boulder, Colorado 80307-3000
email: kennison@ucar.edu

Table of Contents

INTRODUCTION

This document describes an NCAR Graphics package called CONPACKT that allows a user to construct contour plots from data on triangular meshes. CONPACKT provides a sort of tool kit of FORTRAN subroutines that can be called in various combinations to draw different kinds of contour plots.

This section is intended to give an overall view of CONPACKT and selected aspects of its design; it covers some details, but, in general, one should refer to the sections "SUBROUTINES" and "PARAMETERS" for detailed descriptions of subroutines and parameters mentioned. (Parameters are mentioned by name; all the names are of the form 'XXX', where XXX is a three-character mnemonic.) The section "ERROR HANDLING" describes error messages written by CONPACKT. The section "EXAMPLES" describes the examples available for CONPACKT.

It is assumed that the reader is familiar with NCAR Graphics in general and has some knowledge of the packages AREAS and EZMAP/EZMAPA.

Triangular Mesh Structure

Note: See the example "ctex01" (both the code and the first frame that it produces) for help in understanding the structure of a triangular mesh.

To represent a triangular mesh requires three singly-dimensioned arrays: RPNT defines points, IEDG defines edges (each of which consists of two connected points), and ITRI defines triangles (each of which consists of three connected edges). The elements of each array form "nodes" having nominal lengths as follows:

      PARAMETER (LOPN=4)  !  length of a point node
      PARAMETER (LOEN=5)  !  length of an edge node
      PARAMETER (LOTN=4)  !  length of a triangle node
(In some cases, additional elements are needed in some types of nodes, but these are the basic ones that must be present.)

The four elements of a point node are reals, as follows:

  1. the X coordinate of the point;
  2. the Y coordinate of the point;
  3. the Z coordinate of the point;
  4. the field value at the point;
The five elements of an edge node are integers, as follows:

  1. the base index, in RPNT, of the node defining point 1 of the edge;
  2. the base index, in RPNT, of the node defining point 2 of the edge;
  3. the base index, in ITRI, of the node defining the triangle to the left of the edge, plus the index (1, 2, or 3) of the edge within that triangle node (-1 if there is no triangle to the left);
  4. the base index, in ITRI, of the node defining the triangle to the right of the edge, plus the index (1, 2, or 3) of the edge within that triangle node (-1 if there is no triangle to the right);
  5. a utility flag for use by algorithms that scan the structure.
The four elements of a triangle node are integers, as follows:

  1. the base index, in IEDG, of the node defining edge 1 of the triangle;
  2. the base index, in IEDG, of the node defining edge 2 of the triangle;
  3. the base index, in IEDG, of the node defining edge 3 of the triangle;
  4. a flag set non-zero to block use of the triangle, effectively removing it from the mesh. For simple purposes, the values 0 and 1 suffice; however, see the routines CTTDBF and CTTDBM and the internal parameters 'TBX' and 'TBA' for a description of a scheme allowing one to selectively block triangles by using different bits of this flag.
The "left" and "right" sides of an edge are defined as they would be by an observer standing on the front side of the surface defined by the triangular mesh, at point 1, and looking toward point 2, of the edge. It is possible, if there are "holes" in the mesh, that there will be no triangle on the left or on the right of an edge, but there must be a triangle on one side or the other.

Note: The edges pointed to by a particular triangle node must be given in counter-clockwise order, as viewed from the chosen "front" side of the mesh. In fact, it is the ordering of the nodes that defines which side is the "front" and which side is the "back". In the case of a sphere, one would probably use the outside of the sphere as the "front". In the case of a Moebius strip (which I have experimented with a bit), there is a problem with this - you either have to have a seam across the strip or go around it twice to avoid having a seam - but that's probably not a case of great practical interest ... :-)

The "base index" of a point node, an edge node, or a triangle node is always a non-negative multiple of the length of the node, to which can be added an offset to get the index of a particular element of the node. For example, if IPTT is the base index of a triangle of interest, ITRI(IPTT+1) is its first element, which is the base index of the triangle's first edge. Thus, IEDG(ITRI(IPTT+1)+2) is the second element of the triangle's first edge; that is to say, it is the base index of the second point of the first edge of the triangle with base index IPTT. In a similar fashion, it may be seen that RPNT(IEDG(ITRI(IPTT+1)+2)+3) is the third (Z) coordinate of the second point of the first edge of the triangle with base index IPTT.

It is the pointers from the edge nodes back to the triangle nodes that allow CONPACKT to navigate the mesh, moving from triangle to triangle as it follows a contour line. These pointers are a little tricky to define: if IPTE is the base index of an edge node and IEDG(IPTE+3) is zero or more, saying that there is a triangle to the left of the edge, then IEDG(IPTE+3) is the actual index of that element of the triangle node that points to the edge node; that is, ITRI(IEDG(IPTE+3))=IPTE. The base index of the triangle node defining that triangle is IPTT, where IPTT=LOTN*((IEDG(IPTE+3)-1)/LOTN), and the index of the pointer to the edge within the triangle node is IPTI=IEDG(IPTE+3)-IPTT, so that ITRI(IPTT+IPTI)=IPTE. Similar comments apply to element 4 of an edge node, which points into the triangle node defining the triangle to the right of the edge.

For some types of triangular mesh, the maximum number of points, edges, and triangles can be computed easily:

Once we know, at most, how many points, edges, and triangles we're going to have, we can set parameters defining the space to be reserved for the triangular mesh:

      PARAMETER (MPNT=MNOP*LOPN)  !  space for points
      PARAMETER (MEDG=MNOE*LOEN)  !  space for edges
      PARAMETER (MTRI=MNOT*LOTN)  !  space for triangles
Then, we can declare the arrays to hold the point nodes, edge nodes, and triangle nodes defining the triangular mesh:

      DIMENSION RPNT(MPNT),IEDG(MEDG),ITRI(MTRI)
In all of the examples, code like that above (throughout the section) will be found.

Types of Triangular Meshes

The triangular meshes that I have dealt with and provide examples for might be classified as follows:

In the plane:

"ctex01" and "ctterr": These are the examples to look at if you want to learn the details of constructing a triangular mesh from a rectangular grid in the plane completely from scratch. "ctex01" uses a small grid (7 x 7, by default), while "ctterr" is an adapted version of the code that uses a rectangular grid contributed by a user, representing a vertical slice through an ocean basin, and deformed to follow the terrain of the ocean bottom; this grid is dimensioned 4000 x 62, so the triangular mesh consists of 3999 x 61 x 2 = 487,878 triangles, which is a pretty good workout for CONPACKT. Both examples contain a nice little routine, called DRWMSH, to draw the mesh, but, in the interest of making the metafile a bit smaller, the call to it in "ctterr" has been commented out. "ctex01" also contains code to label the points, edges, and triangles of the mesh, but these have been omitted from "ctterr".

"ctex02": Demonstrates a possible way to construct, in the plane, a triangular mesh that fills a particular area of interest (in this case, the contiguous "lower 48" states of the US). Again, this example constructs the triangular mesh completely from scratch; it begins with a set of equilateral triangles that completely fill the map space, discards those that are wholly outside the area of interest, and adapts the others to better fit that area.

In three dimensions, on the globe, rectangular:

Each of these examples uses EZMAP routines to show the earth in orthographic projection, as seen from four different viewpoints, and each calls the routine CTTMRG to deal with a rectangular grid mapped onto the surface of the globe. In the code for any of these examples, search for the call to CTTMRG to see how the triangular mesh is constructed.

"ctllg1": In this example, the grid is one created for illustrative purposes; it is dimensioned 81 x 41 and is mapped onto the portion of the globe between longitudes 80W and 80E and latitudes 40S and 40N, so that it covers a limited portion of the globe.

"ctllg2": In this example, the grid is one created for illustrative purposes; it is dimensioned 171 x 81 and is mapped onto the portion of the globe between longitudes 170W and 170E and latitudes 80S and 80N, so that it nearly covers the globe.

"ctllg3": In this example, the grid is one created for illustrative purposes; it is dimensioned 181 x 91 and is mapped onto the entire globe. All of the points along the top edge of the rectangular grid map to the North Pole and all the points along its bottom edge map to the South Pole; the left and right edges of the rectangular grid meet along a seam that runs from the North Pole to the South Pole along the International Date Line.

"ctgaus": This example uses a Gaussian grid from Paul Swarztrauber; it is dimensioned 129 x 257 and is rotated 90 degrees as compared to the grids of examples "ctllg1", "ctllg2", and "ctllg3". It is mapped onto most of the globe. The left and right edges of the grid map to little circles around the North and South poles, respectively, while the top and bottom edges of the grid meet along a seam that runs along a meridian from pole to pole.

"ctpopg": This example uses a typical POP grid from a user site; it is dimensioned 101 x 116 and is mapped onto the entire globe, less two little circles. Think of this grid as being wrapped around the globe so that its left and right edges meet along a seam on a meridian and then elastically deformed so as to pull the little circle formed by its top edge to a position over Greenland, while leaving the little circle formed by its bottom edge centered over the South Pole and wholly contained within Antarctica. Finally, portions of the grid over land are omitted, as this grid is used for ocean modelling.

"ctorca": This example uses a typical ORCA grid from a user site; it is dimensioned 181 x 148 and is mapped onto the entire globe, less a circle at the South Pole and a few other areas over Asia and Africa. Think of wrapping this grid around the globe so that its left and right edges meet along a seam on a meridian and then squashing flat the circle formed by its top edge in such a way that it becomes an extension of the left/right seam passing through the North Pole. (The circle formed by the bottom edge of the grid is centered on the South Pole and remains open.) Certain rectangular portions of the grid are then severed from the rest of it and shifted elastically so as to provide denser coverage of certain areas (the Mediterranean, Black, Caspian, and Red Seas). Finally, portions of the grid over land are omitted, as this grid is used for ocean modelling.

"ctfite": This example uses a POP grid from a user (I got it from Fred Clare); it is dimensioned 321 x 384 and is mapped onto the globe in much the same way as the grid of "ctpopg". The data are actual user data and show ice thickness, so there is little of interest except at the poles.

"ctswth": This example uses a rectangular grid from Simona Bordoni and represents the area seen by a satellite during one orbit of the earth. I think the data are wind speeds over the ocean.

In three dimensions, on the globe, triangular:

Each of these examples uses EZMAP routines to show a portion of the earth in orthographic projection. Each calls the routine CTTMTL to deal with an intrinsically triangular mesh mapped onto the surface of the globe. In the code of any of these examples, search for the call to CTTMTL to see how a triangular mesh of the form expected by CONPACKT is constructed from triangles provided by a user.

"ctcbay": This example uses data provided by Tom Gross. It consists of the latitudes and longitudes of 7,258 points on the globe, plus an array of 13,044 index triplets, each specifying the indices of three of those points forming a triangle. The triangular mesh represents Chesapeake Bay.

"ctnccl": This example uses data provided by Brett Estrade. It consists of the latitudes and longitudes of 32,218 points on the globe, plus an array of 58,641 index triplets, each specifying the indices of three of those points forming a triangle. The triangular mesh represents the ocean off the coast of North Carolina.

In three dimensions, on the globe, geodesic:

Each of the examples "ctgeo1", "ctgeo2", and "ctgeo3" uses EZMAP routines to show the earth in orthographic projection, as seen from four different viewpoints, and each uses a geodesic grid constructed using a different method. In all three cases, the net effect is to subdivide the faces of an icosahedron (a regular Platonic solid with twenty equilateral triangles as faces), into smaller triangles and then project the vertices of those triangles outward onto the surface of a circumscribed sphere. Subdivision of a triangle is done using N-1 lines parallel (in some cases, roughly parallel, I think) to each of its three sides, dividing each side into N pieces; by default, N = 16, but, in "ctgeo1" and "ctgeo2", this is set by a PARAMETER statement and so is easily changed. Each face is thereby subdivided into N**2 (by default, 256) smaller triangles, so the total number of triangles is 20*N**2 (by default, 5120). The number of edges is 30*N**2 (by default, 7680) and the number of vertices is 10*N**2+2 (by default, 2562).

"ctgeo1": In this example, the geodesic grid is constructed in a clever way that makes very efficient use of memory, but the code to do it, in the subroutine GTGEO1, will probably prove quite difficult to follow. Each triangular face of the icosahedron is subdivided, in a single step, into N**2 smaller triangles of the same size. Once those triangles are projected outward onto the sphere, some of them (those near the center of a face of the icosahedron) are quite a bit larger than others (those near a vertex of the icosahedron).

"ctgeo2": In this example, two innovations were introduced in the subroutine GTGEO2, which constructs the geodesic grid:

"ctgeo3": This example makes use of data from the NetCDF file "C02562.global.nc", which was downloaded from the following Web site:

Go to "Step 2", click on "sample spherical geodesic grids", and then on "Geodesic grid with 2562 cells". (Actually, "ctgeo3" can easily be modified to use either of the other grids mentioned there, as well.)

These data are of interest in two ways:

"ctgc23": This example produces four plots comparing the geodesic grids of examples "ctgeo2" and "ctgeo3". Two of the plots show the grids superimposed (on a hemisphere of the globe and in a relatively close-up view); the other two plots show histograms of the areas of the triangles produced by the two methods.

In three dimensions, on the globe, other:

"ctiscp": This example uses a grid created by the "International Satellite Cloud Climatology Project" (ISCCP). It consists of 6596 cells on the globe, each of which is a rectangle on a cylindrical equidistant projection. The cells are created by dividing the surface of the earth into strips, parallel to the equator, each of which is 2.5 degrees of latitude wide, and then subdividing each strip into an integral number of cells in such a way that all of the cells have almost the same surface area on the globe. The subroutine GTISCP triangulates this grid by connecting the centers of "adjacent" cells and then using the connecting lines to form triangles. The code is rather difficult to describe and may be a bit difficult to read.

"ctisc2": This example represents an attempt to deal with the ISCCP grid in a more understandable way. It treats each cell as a polygonal patch like those of example "ctgeo3" and then uses the technique of that example to determine which of the polygonal patches are adjacent. (Some of the polygonal patches have one or more sides of zero length.) Whether the resulting code is easier to understand than that of "ctiscp" is debatable.

"ctwng1" and "ctwng2": These examples use SEAM grids (where SEAM stands for "Spectral Element Atmospheric Model") obtained from Houjun Wang. See the following Web site for more informataion about such grids:

The basic elements of each grid are quadrilateral patches on the surface of the globe, obtained by subdividing the faces of a cube and projecting the pieces outward onto the surface of the globe. The patches are further subdivided into smaller quadrilaterals; in "ctwng2", in particular, this is done in such a way as to provide higher-resolution coverage of the US. Each of the quadrilaterals is then split in half along a diagonal to form two triangles and the routine CTTMTL or CTTMTX is used to form a triangular mesh of the form expected by CONPACKT routines.

In three dimensions, on an arbitrary surface:

"cttd01" and "cttd02": These examples demonstrate that CONPACKT is not limited to dealing with triangular meshes in a plane or on the surface of the globe. In each case, the triangular mesh used represents an arbitrary surface in 3-space (looking, perhaps, a bit like one of the dancing mushrooms from Disney's "Fantasia"); one of the meshes forms a closed object, while the other has a hole in it through which the "back" side of the mesh is visible. The 3D package TDPACK is used to draw perspective views of these grids, with contours on them.

The routine CTTDCA is used to create a cell array that creates an accurate "picture" of the object. The routine CTTDBF is used to set bits in the blocking flags for the triangles to reflect various conditions (triangle hidden behind another, triangle seen from the back side, triangle seen nearly edge-on) and CTTDBM is used, just before drawing the mesh itself or just before drawing contours on the mesh, to allow drawing in only those triangles that, for example, are seen from the front, are not hidden, and are not too nearly edge on. The net result is to solve the "hidden-line" problem for the mesh or for the contours on a triangle-by-triangle basis (not quite as good as one would like, but not too bad, either).

Routines Which May Be Called

The following routines are meant to facilitate the process of creating a triangular mesh of data:

Once a triangular mesh has been defined, the code to draw a contour plot will probably begin with several calls to set internal parameters affecting the behavior of the routines called after that. All the internal parameters have default values; only those which are to have values different from the default need to be set. Routines which may be called to set the values of parameters are as follows:

In general, once a parameter is given a value by a call to one of these routines, it retains that value until a similar call resets it. Thus, many of the parameter-setting calls need to be done only once and do not need to be repeated for each new contour plot.

After all required parameters have been set, the process of drawing a contour plot begins with a call to an initialization routine:

This routine CTMESH performs initialization required for subsequent calls to work properly. The dimensions of the arrays defining the triangular mesh and the dimensions of the real and integer workspace arrays are copied to internal variables. Pointers in the array defining the edges of the mesh are adjusted so as to make the data value at the first point of each edge less than or equal to the data value at the second point of each edge, which is important for various algorithms in other routines. Pointers used to manage the real and integer workspaces are initialized. It is decided how the 3D coordinates of the points of the triangular mesh are to be mapped into 2D user coordinates and how those coordinates are to be mapped to the plotter frame. If the user has not called the SPPS routine SET (or done the equivalent GKS calls), that call is done. The minimum and maximum values in the data array are found and it is decided whether or not the data field is essentially constant. If contour levels are to be chosen automatically, the parameter arrays specifying the contour levels are cleared, so that automatic selection will take place when these levels are required.

Among the internal parameters are arrays completely specifying contour levels of interest and what is to be done at each of those levels. These arrays may be used to take complete control of the contouring process; most users will probably elect not to do this, but to let CONPACKT choose the levels. At this point, then, as a rule, none of these parameter arrays will have been filled. No calls need be done to fill them; if they are empty when they are needed, the required values will be chosen at that point. For certain applications, however, it is desirable to force the selection of contour levels and perhaps the character strings which are to be used as contour labels. This may be done by means of calls to one or both of the following routines:

The advantage of calling one or both of these routines is that, after each call, one can modify what the routine called has done in order to produce desired effects. For example, after CTPKCL is called, one might set elements in parameter arrays which will cause the labelled contour lines to be solid and the unlabelled contour lines to be dashed. As another example, after CTPKLB is called, one might check for the string "0" as a label and change it to "0.0".

At this point, various other routines may be called:

Four routines may be called when 'MAP' is set to 2, which says that the 3D package TDPACK is being used to project the triangular mesh into the plane.

Finally, to advance the frame, the user must call the SPPS routine FRAME; CONPACKT won't do it.

At any time, it is possible to retrieve the value of an internal parameter by calling one of the three following routines:

Several routines in CONPACKT are not called by the user, but by CONPACKT itself. The default versions of these routines, in all cases but one, do nothing; the routines exist simply to be replaced by the user. These routines are as follows:

Two additional routines are provided for use in error-recovery situations:

Different Styles of Contour Plots

The design of CONPACKT allows one to construct contour plots in many different styles. Which style is chosen will depend on the amount of computer time and memory available, on the capabilities of the device on which the plots are being drawn, and on the intended use of the plots. Some possibilities are discussed below.

If the intent is to draw the contour plot using relatively few computer resources, the following sequence of calls will suffice:

  1. Call parameter-setting routines.

  2. Call CTMESH to initialize the drawing of the contour plot.

  3. Call CTBACK to draw a simple background.

  4. Call CTCLDR to draw the contour lines, with labels generated by a dashed-line package.

  5. Call CTLBDR to draw an informational label below the plot and high/low labels on the plot.

Assuming that step 1 is null and that all default parameter values are used, the resulting plot will look much like what would have been drawn by CONREC. The labels won't be positioned very well and lines may be drawn through them. The characters used for the informational label and for high and low labels will be of better quality than those used for the line labels; if desired, additional resources may be saved by a parameter-setting call forcing the PLOTCHAR package to use lower-quality characters.

To produce a better-positioned set of labels, step 1 above should include a call to CTSETI setting the parameter 'LLP', which says how line labels are to be positioned, to one of the values 3 or -3; this turns off the generation of line labels by a dashed-line package called by CTCLDR and causes them to be positioned and written by the routine CTLBDR instead, using a penalty scheme which produces quite pleasing results. Contour lines will still pass through line labels positioned in this way.

Contour lines may be prevented from passing through labels drawn by CTLBDR in one of two ways, depending on the nature of the plotting device. If the device allows for the use of the GKS fill area primitive and if the result of drawing one object on top of another is that the pixels affected by drawing the second object simply change color (as happens on most terminals, but not usually on a device which exposes film, for example), then one may insert calls changing the values of the parameters 'ILB', 'HLB', and 'LLB' in such a way as to force boxes surrounding the informational label, the high and low labels, and the line labels to be filled with the background color prior to the drawing of the labels. This has the effect of preventing the contour lines from passing through the labels.

If filling the label boxes will not work, then a software technique, using routines in the utility AREAS, may be used instead. The sequence of calls will then be as follows:

  1. Call parameter-setting routines.

  2. Call CTMESH to initialize the drawing of the contour plot.

  3. Call CTBACK to draw a simple background.

  4. Call ARINAM (in AREAS) to initialize an area map.

  5. Call CTLBAM to generate a list of label positions and to put label boxes for the labels at those positions into the area map.

  6. Call CTCLDM to draw contour lines masked against the area map. Each line is broken into pieces lying outside label boxes and pieces lying inside label boxes and only the former are drawn.

  7. Call CTLBDR to draw the labels in the boxes left vacant by step 6.

Some users may wish to draw a solid-filled contour plot, rather than a line plot. This is also done using routines in the utility AREAS, as follows:

  1. Call parameter-setting routines.

  2. Call CTMESH to initialize the drawing of the contour plot.

  3. Call ARINAM (in AREAS) to initialize an area map.

  4. Call CTCLAM to put contour lines into the area map.

  5. Call ARSCAM (in AREAS) to scan the area map and to recover from it the polygons created by the lines in the area map. A user-defined routine is called to fill (or not to fill) each polygon. Filling may be done by calls to routines in the utility SOFTFILL or to the GKS routine GFA.

Labels may be written on a solid-filled contour plot; this requires adding a call to CTLBAM after step 4 and a call to CTLBDR after step 5. It may be better, though, to draw a key for the solid-filled plot. There is no routine in CONPACKT to do this, but the NCAR Graphics utility LABELBAR provides such a capability.

The packages AREAS, CONPACKT, and EZMAP/EZMAPA may be used cooperatively to achieve other desired effects. For example, if the contour plot being drawn represents output from an ocean model, it may be desirable to draw contours (or to fill contour bands) only over the oceans on a background drawn by EZMAP.

A solid-filled contour plot may be drawn in another way, using the GKS primitive "cell array". The steps required are as follows:

  1. Call parameter-setting routines.

  2. Call CTMESH to initialize the drawing of the contour plot.

  3. Call CTCICA to generate color indices in a cell array.

  4. Call the GKS routine GCA to "paint" the cell array.

Using cell arrays has advantages and disadvantages compared to using AREAS to generate GKS "fill areas". One disadvantage is that, for the same sort of quality, a rather high-resolution cell array must be used; it may be time-consuming and space-consuming to generate and to display such a cell array; in particular, using the "zoom" capability of "idt" on a cell array doesn't work too well. Another disadvantage of the cell-array approach is that it is more difficult (sometimes impossible) to limit the coloring of contour bands to specific areas (as, for example, over land or over ocean), which can be done relatively easily using the fill-area approach. The major advantage of the cell-array approach is that it avoids the problems that, to some extent, accompany the use of the package AREAS. (While many of these problems have been fixed, one can still expect an occasional glitch, particularly when using certain EZMAP projections.)

Contour Level Selection

The routines CTCLAM, CTCLDM, CTCLDR, CTLBAM, and CTLBDR all generate output for specified sets of contour levels. The parameter 'NCL' specifies the number of different contour levels for which something is to be done by one or more of the above routines. The value of 'NCL' may not exceed 256. For each value of I from 1 through 'NCL', the Ith element of the parameter array 'CLV' specifies a contour level and the Ith element of each of the parameter arrays 'AIA', 'AIB', 'CLC', 'CLD', 'CLL', 'CLU', 'LLC', and 'LLT' is an associated datum, saying something about what is to be done at that contour level. Detailed descriptions of these parameter arrays are given in the section "PARAMETERS", below; thumbnail descriptions of elements of these parameter arrays are as follows:

The contents of these parameter arrays may be specified completely by CONPACKT, completely by the user, or some of both. The parameter 'CLS' says whether or not CONPACKT is to select contour levels and associated quantities and, if so, in what manner. The default value of 'CLS' indicates that CONPACKT is to select about 16 contour levels at multiples of some nice interval, which it is to choose. By default, then, the call to CTMESH zeroes 'NCL'; during the first subsequent call which requires contour levels to have been chosen, they are chosen. The associated parameter elements are set to indicate which contour lines should be labelled, what character strings should be used as labels, which lines should be put in an area map by a call to CTCLAM, and what area identifiers should be used for areas "above" and "below" these lines.

Other values of 'CLS' may be used to change the way in which CONPACKT chooses contour levels. See the detailed descriptions of 'CLS' and of the other parameters 'CIS', 'CIT', 'CIU', 'CMN', 'CMX', 'LIS', 'LIT', and 'LIU'.

The user may elect to set all of the contour levels and associated quantities. Suppose, for example, that it is desired to draw labelled solid lines for each of the values .1, .2, .3, ..., .9 and unlabelled dashed lines for each of the values .05, .15, .25, ... .95. The following code, inserted before the call to CTMESH, will set the required parameters:

      CALL CTSETI ('CLS - CONTOUR LEVEL SELECTION',0)
      CALL CTSETI ('NCL - NUMBER OF CONTOUR LEVELS',19)
      DO 101 I=1,19
        CALL CTSETI ('PAI - PARAMETER ARRAY INDEX',I)
        CALL CTSETR ('CLV - CONTOUR LEVEL VALUE',REAL(I)/20.)
        IF (MOD(I,2).EQ.1) THEN
          CALL CTSETI ('CLU - CONTOUR LEVEL USE',1)
          CALL CTSETI ('CLD - CONTOUR LINE DASH PATTERN',21845)
        ELSE
          CALL CTSETI ('CLU - CONTOUR LEVEL USE',3)
          CALL CTSETI ('CLD - CONTOUR LINE DASH PATTERN',65535)
        END IF
  101 CONTINUE
In the above code, 'CLS' is zeroed to suspend the selection of contour levels by CONPACKT itself. Then, 'NCL' is set to say how many contour levels are to be defined. Then, in a loop on I from 1 to 19, 'PAI' is set to tell CONPACKT which element of each parameter array is to be set, the Ith element of 'CLV' is set to REAL(I)/20., which, for each I, gives one of the desired contour levels, the Ith element of 'CLU' is set to a 1 if just the line is to be drawn or to a 3 if both the line and the labels for the line are to be drawn, and the Ith element of 'CLD' is set to 21845 (octal 52525) if a dashed line is to be used or to 65535 (octal 177777) if a solid line is to be used.

Note that 'NCL' must be set prior to setting any element of 'CLV' or the associated arrays.

Note also that, when an element of 'CLV' is set, all of the associated elements of the associated arrays receive a default value. (In fact,the default element of 'CLU' is 1, and the default element of 'CLD' is a pattern specifying a solid line, so two of the calls in the code above are redundant.)

Note also that the use of CTSETI to specify the value of 'CLD' will work only if 'DPU' is positive, implying the use of DASHCHAR routines; if 'DPU' is negative, DASHPACK routines will be used and, by default, they will expect underscores, rather than apostrophes, to represent gaps, so that CTSETC calls will be needed to define the dash patterns.

In some cases, the user will want to let CONPACKT choose a set of contour levels and then either add other levels of interest, modify elements of the associated parameter arrays, or both. Suppose, for example, that it is desired to have CONPACKT pick the levels, that contour lines at positive levels are to be drawn in red, that contour lines at negative levels are to be drawn in blue, and that contour lines at the zero level are to be drawn in white. The following code, inserted after the call to CTMESH, would do the job (assuming that color indices IBLU, IRED, and IWHI have previously been defined):

      CALL CTPKCL (...)
      CALL CTGETI ('NCL - NUMBER OF CONTOUR LINES',NOCL)
      DO 101 I=1,NOCL
        CALL CTSETI ('PAI - PARAMETER ARRAY INDEX',I)
        CALL CTGETR ('CLV - CONTOUR LEVEL VALUE',CLEV)
        IF (CLEV.LT.0.) THEN
          CALL CTSETI ('CLC - CONTOUR LINE COLOR INDEX',IBLU)
        ELSE IF (CLEV.GT.0.) THEN
          CALL CTSETI ('CLC - CONTOUR LINE COLOR INDEX',IRED)
        ELSE
          CALL CTSETI ('CLC - CONTOUR LINE COLOR INDEX',IWHI)
        END IF
  101 CONTINUE
In the code above, the routine CTPKCL is called to force CONPACKT to pick a set of contour levels. Then, the value of 'NCL' that it chose is retrieved, and a loop is run from 1 to that value. On each pass through the loop, the parameter array index 'PAI' is set to tell CONPACKT what element of the parameter arrays is being accessed and then one of the contour levels chosen is retrieved to the variable CLEV. Depending on the value of CLEV, the associated element of the parameter array which specifies the color index of the contour lines at that level is set to produce a blue line, a red line, or a white line.

CONPACKT Coordinate Systems

The mapping of contours into the plotter frame depends on three things:

By default, to describe contour lines and other objects on the contour plot, CONPACKT generates X, Y, and Z coordinates representing points on the edges of the triangles defined by the data of the triangular mesh and then discards the Z coordinate, using only the X and Y coordinates as the user coordinates in calls to NCAR Graphics routines.

If the internal parameter 'MAP' is given a positive non-zero value, each triplet of X, Y, and Z coordinates is mapped, prior to use, by a statement of the form

      CALL CTMXYZ (IMAP,XINP,YINP,ZINP,XOTP,YOTP)
IMAP is the value of 'MAP'; XINP, YINP, and ZINP are the unmapped (input) 3D coordinates; and XOTP and YOTP are the mapped (output) 2D coordinates to be used as "user coordinates".

The default version of CTMXYZ does the following mappings (where RTOD = 57.2957795130823 (180/pi):

The subroutine CTMXYZ may be replaced by a version which does other desired mappings. If the CONPACKT routines are loaded from a binary library, this can usually be done by just compiling one's own version of the routine, so that it replaces the one from the library. The definition of mapping 1 should not be changed.

The way in which "user coordinates" are mapped to "fractional coordinates" in the plotter frame is determined by the current definitions of the "window" in the user system and the "viewport" on the plotter frame. The window and viewport may have been defined by a call to the SPPS routine SET or by calls to GKS routines; the former will be described.

A call to the SPPS routine SET has the form

      CALL SET (XVPL,XVPR,YVPB,YVPT,XWDL,XWDR,YWDB,YWDT,LNLG)
All arguments are REALs except for LNLG, which is an INTEGER. The first four arguments must all be between 0 and 1, inclusive; they define a rectangular area in the fractional coordinate space of the plotter frame known as the "viewport". The next four arguments define a rectangular area in "user coordinate" space known as the "window". The final argument indicates whether the mapping of user coordinates into the viewport is to be linear or logarithmic in X and Y. See the documentation of the package SPPS for further details.

By default, CONPACKT (specifically, the routine CTMESH) calls SET. One may, by setting the parameter 'SET' to zero, prevent CONPACKT from doing this; in that case, one must do the call for oneself or depend on some other utility (such as EZMAP) to have done it.

If CONPACKT calls SET, it always uses LNLG = 1, requesting a linear-linear mapping from the window to the viewport, and it positions the viewport and window as follows: The viewport is positioned as specified by the current values of the parameters 'VPL', 'VPR', 'VPB', 'VPT', and 'VPS'. The first four of these specify the position of a "viewport area", in which the viewport is to be centered and made as large as possible; the final one says how the shape of the viewport is to be determined. By default, the position of the window in the user coordinate system is determined by computing the minimum and maximum values of X and Y over all the points of the triangular mesh. The parameters 'WDL', 'WDR', 'WDB', and 'WDT' may be used to override this default behavior and specify the exact values to be used in the SET call to define the window.

If the triangular mesh represents data on the surface of a globe of radius 1, then to map the CONPACKT output onto an EZMAP background, one need only set 'MAP' to 1 and initialize EZMAP (which results in a call to the SPPS routine SET).

Special-Value Areas

CONPACK had a parameter 'SPV', whose value could be used in a data array in place of missing or unreliable data: any cell with this value at one or more of its vertices was essentially omitted from the rectangular grid. CONPACKT has no such parameter, but there are two ways to achieve the same effect:

There is no effective difference between triangles that are actually omitted from the mesh and triangles that are left in the mesh and marked as "blocked". The routine CTCLAM adds to an area map edges having omitted or blocked triangles on one side of them. The routines CTCLDM and CTCLDR may be made to draw edges having omitted or blocked triangles on one side of them (by giving a non-zero value to element "-1" of the parameter array 'CLU').

The Out-of-Range Parameter and Out-of-Range Areas

The parameter 'ORV', if non-zero, specifies an "out-of-range" value. This is only of use when the parameter 'MAP' is non-zero, specifying that coordinates are to be mapped by calling the subroutine CTMXYZ. The X coordinate returned by CTMXYZ may be set equal to 'ORV' to indicate that the mapped point is outside the range in which the mapping is defined.

A possible value for 'ORV', if it is to be set non-zero, is 1.E12, which has historically been returned by the EZMAP routines MAPTRN and MAPTRA to indicate a point which is outside the area depicted by a given map projection.

The union of all points for which CTMXYZ returns the out-of-range value constitutes a set of out-of-range areas. Contour lines are not traced in out-of-range areas (indeed, they cannot be). A binary-halving technique is used to extend contour lines to the very edge of such areas. The routine CTCLAM will attempt to generate and add to the area map a set of edges for such areas, and the routines CTCLDM and CTCLDR may be made to attempt to draw the edges of such areas (by giving a non-zero value to element "-2" of the parameter array 'CLU').

When contour lines are traced, if two consecutive points are out of range (in range), then the entire line segment connecting those two points is assumed to be out of range (in range). If the detail of the out-of-range areas is small enough, this assumption may cause errors. Giving the parameter 'PIC' a non-zero value will cause more points to be examined along each such line segment, thus curing the problem. For similar reasons, the algorithms used to trace the edge of the grid, the edges of special-value areas, and the edges of out-of-range areas may fail. Giving the parameter 'PIE' a non-zero value will cause these algorithms to use a finer grid, thus, again, curing the problem.

2D Smoothing of Contour Lines

Each of the routines which generates contour lines (CTCLAM, CTCLDM, and CTCLDR and the internal routine which positions labels along the lines using either the penalty scheme or the regular scheme) may be caused to smooth those lines, using cubic splines under tension. Giving the parameter 'T2D' a non-zero value causes this smoothing to be done. The absolute value of 'T2D' specifies the tension to be used; values near zero (.001, for example) yield approximately cubic splines (which may give very "loopy" curves), and large values (15., for example) yield nearly polygonal curves. Using very large values (50., for example) can cause overflow in the routines that do the smoothing.

Note that, since each contour line is smoothed separately, there is no way to absolutely ensure that it will not cause adjacent contour lines to cross each other; one must experiment with the tension to reduce the probability of that to a minimum. A reasonable value to start with is 2.5.

If 'T2D' is negative, smoothing is done prior to the coordinate mapping, if any, implied by the setting of the parameter 'MAP'. If 'T2D' is positive, smoothing is done after the mapping.

The parameter 'PIC' says how many points are to be interpolated between each pair of points defining the contour line, before smoothing. If 'PIC' is given a non-zero value when the 2D smoother is turned on, the effect is to constrain the smoothed curves to more closely approximate the original polygonal lines.

The parameter 'SSL' specifies the distance between points used to draw the smoothed contour lines. It is expressed as a fraction of the width of the window in the coordinate system in which the smoothing is being done.

Dash Package Use by CONPACKT

By default, CONPACKT uses routines from the old dash package called DASHCHAR. By using the appropriate flags on the "ncargf77" command line (or, if "ncargf77" is not being used, by specifying a different set of libraries on a link/load command), one can substitute one of the other members of the family (DASHSMTH or DASHSUPR).

One can also tell CONPACKT to use routines from a new dash package, called DASHPACK, which is intended to replace all of the older packages. One does this by giving the internal parameter 'DPU' a negative value in place of the default positive value. The new dash package offers many advantages over the older ones; for a full description of it, see the programmer document called "DASHPACK, A SOFTWARE PACKAGE FOR DRAWING DASHED LINES".

When DASHPACK is used, the following considerations apply:

Hachuring of Contour Lines

The parameters 'HCL', 'HCF', and 'HCS' may be used to request that contour lines be "hachured" in one of various ways. "Hachuring" is the process of drawing "hachures": short line segments perpendicular to the contour and usually drawn on the "downslope" side of it.

Note: When hachuring is activated, it may be necessary to increase the value of the internal parameter named 'RWC'. See the descriptions of the parameters 'HCF' and 'RWC' for more information.

Labels

Two or three different types of labels are written by a call to the routine CTLBDR: an informational label, high and low labels, and contour-line labels (the latter only if ABS('LLP') is greater than 1). Boxes surrounding these labels may be added to an area map by calling the routine CTLBAM; the purpose of this will probably be to prevent contour lines drawn by a subsequent call to the routine CTCLDM from passing through the labels or to prevent the label boxes from being filled or colored by a subsequent call to the routine ARSCAM (in the package AREAS).

When a constant field was detected by the initial call to CTMESH, a fourth type of label may be written by CONPACKT routines. In this case, a call to CTLBDR will write a constant-field label, warning of the situation, in place of the labels it would normally write. A call to CTLBAM will add the label box for the constant-field label to the area map, instead of the label boxes for the other labels. Calls to CTCLDR and CTCLDM which would normally draw contour lines will write the constant-field label instead.

The appearance of all of these labels may be determined in detail by setting parameters:

In all of the above parameter names, a suffixed 'A' means "angle", 'B' means "box flag", 'C' means "color index", 'L' means "line width", 'S' means "size of characters", 'T' means "text of label", 'W' means "white space width", 'X' means "X coordinate", and 'Y' means "Y coordinate". The 'O' in 'HLO' means "overlap", while the 'O' in 'LLO' means "orientation".

All labels are written by means of calls to the character-plotting routine PLCHHQ, in the package PLOTCHAR. The angle, in degrees, at which a label is written is determined by the value of the parameter 'xxA' (and, if it is a contour-line label, by the value of the parameter 'LLO'). The box flag 'xxB' determines whether or not, prior to writing the label, a box surrounding it is filled, and whether or not, after writing the label, the edge of the box is drawn. If the box is filled, it is done using the color index specified by the parameter 'LBC'; if the edge of the box is drawn, it is done using the color index, if any, chosen for the label itself, which is determined by the value of the parameter 'xxC'. The line width to be used in drawing the box is determined by the value of the parameter 'xxL'. The size (width) of the characters is determined by the value of the parameter 'xxS'. The text of the label is determined by the value of the parameter 'xxT'; usually, this string may contain embedded substrings of the form '$xxx$', which are to be replaced by the value of the quantity specified by the three-character mnemonic 'xxx'. The width of the "white space" to be left around the label (which defines the dimensions of the box around it) is determined by the value of the parameter 'xxW'.

Positioning of Labels on Contour Lines

CONPACKT provides three different ways to position labels on contour lines. The parameter 'LLP' is set by the user to choose one of the three.

When 'LLP' is 2 or 3, smoothing, if any, implied by a non-zero value of 'T2D' is suspended during placement of contour line labels. This saves a good deal of computer time and space and provides almost as good a set of labels. To leave smoothing turned on during placement of contour line labels, use 'LLP' = -2 or -3.

Details of the Penalty Scheme for Positioning Contour Line Labels

The penalty scheme is controlled by the ten parameters 'PC1', 'PC2', 'PC3', 'PC4', 'PC5', 'PC6', 'PW1', 'PW2', 'PW3', and 'PW4'. (The rationale behind the names is that the first six parameters are characterized as "constants" and the remaining four as "weights" for the four terms in the penalty function.)

A point P on a contour line will be rejected as the center point of a label under any of the following conditions:

  1. If there are fewer than three points on the line.

  2. If the point P is too close to the center point of any other label on the current line, where the meaning of "too close" is defined by 'PC6'.

  3. If a label at the point P would extend outside the current viewport.

  4. If a label at the point P would overlap any previous label.

  5. If the estimated gradient of the field being contoured is too large at the point P, where "too large" is defined by the value of 'PC1', and 'PW1', the weight of the first term in the penalty function, is non-zero.

  6. If the estimated number of contour lines crossing a label at the point P is too large, where "too large" is defined by the value of 'PC2', and 'PW2', the weight of the second term in the penalty function, is non-zero.

  7. If the cumulative change in direction of the contour line within a circle covering a label at the point P is too large, where "too large" is defined by the value of 'PC3', and 'PW3', the weight of the third term in the penalty function, is non-zero.

The penalty function computed at each remaining point has the form

      PFUN = PW1 * GRAD / (GRAV+PC1*GRSD)            GRADIENT TERM
     +       PW2 * ENCB / PC2                        NUMBER-OF-CONTOURS TERM
     +       PW3 * CDIR / PC3                        CHANGE-IN-DIRECTION TERM
     +       PW4 * MIN (1-EXP(-((D(I)-PC4)/PC5)**2)) OPTIMUM-DISTANCE TERM
The first term of the penalty function becomes larger in high-gradient regions. GRAD is the estimated gradient at the point P, GRAV is the average gradient over the whole field being contoured, and GRSD is the standard deviation of the estimated gradients over the whole field. The parameter 'PC1' specifies how far from the norm gradients are allowed to wander, as a multiple of the standard deviation. Condition 5 above implies that, for points at which the penalty function is computed, either 'PW1' is zero or GRAD is less than or equal to GRAV+PC1*GRSD.

The second term of the penalty function becomes larger as ENCB, the estimated number of contour bands crossing a label at the point P, increases. The parameter 'PC2' specifies the largest number of crossing bands allowed. Condition 6 above implies that, for points at which the penalty function is computed, either 'PW2' is zero or ENCB is less than or equal to 'PC2'.

The third term of the penalty function becomes larger as CDIR, the cumulative change in direction of the contour line in a circular region centered at the point P and with a radius equal to half the larger dimension of the label, increases. The parameter 'PC3' specifies the largest such cumulative change allowed, in degrees. Condition 7 above implies that, for points at which the penalty function is computed, either 'PW3' is zero or CDIR is less than or equal to 'PC3'.

The fourth term of the penalty function becomes larger as the distance of the point P from the centers of all labels previously placed on other contour lines deviates from an optimum value specified by the user. D(I) represents the distance to the Ith such label center. The minimum is taken over all values of I. The parameter 'PC4' is the user-specified optimum distance, specified as a fraction of the width of the current viewport. If the point P is exactly 'PC4' units away from some previous label, then "MIN(1-EXP(...))" will have the value 0; otherwise, it will be non-zero. The parameter 'PC5' is the "folding distance", specified as a fraction of the width of the current viewport; as its value decreases, the function "1-EXP(...)" develops a sharper spike at D(I) = 'PC4'.

Thumbnail descriptions and default values of all the user-settable parameters are given below:

    'PC1'=1.   MULTIPLIER OF THE STANDARD DEVIATION OF THE GRADIENTS
    'PC2'=5.   MAXIMUM NUMBER OF CROSSING CONTOUR BANDS
    'PC3'=60.  MAXIMUM CUMULATIVE CHANGE IN DIRECTION OF THE CONTOUR LINE
    'PC4'=.05  OPTIMUM DISTANCE, AS A FRACTION OF THE WIDTH OF THE VIEWPORT
    'PC5'=.15  FOLDING DISTANCE, AS A FRACTION OF THE WIDTH OF THE VIEWPORT
    'PC6'=.30  MINIMUM DISTANCE BETWEEN LABELS ON THE SAME CONTOUR LINE, AS
                   A FRACTION OF THE WIDTH OF THE VIEWPORT
    'PW1'=2.   WEIGHT OF THE GRADIENT TERM
    'PW2'=0.   WEIGHT OF THE NUMBER-OF-CONTOURS TERM
    'PW3'=1.   WEIGHT OF THE CHANGE-IN-DIRECTION TERM
    'PW4'=1.   WEIGHT OF THE OPTIMUM-DISTANCE TERM

Choosing a Scale Factor

It is possible to specify a scale factor by which field values are to be divided before conversion to a character form for display as a numeric label.

The parameter 'SFS' says how the scale factor is to be chosen. If it is given a value greater than zero, that value is the desired scale factor. If 'SFS' is given a value less than or equal to zero, CONPACKT is directed to choose a scale factor to use, in one of five different ways. The value of the parameter 'SFU' may be retrieved by the user; it specifies the scale factor which has been selected for use.

The value of the scale factor may be displayed as a part of the informational label. This is done by embedding the substring '$SFU$' in the string which gives the value of the parameter 'ILT'.

The default value of 'SFS' is 1, which essentially specifies that no scale factor is to be used.

Constant Field Detection

The routine CTMESH checks for a field which is essentially constant. When such a field is found, the parameter 'CFF' is set non-zero; otherwise, 'CFF' is zeroed. The value of 'CFF' may be retrieved by the user.

When 'CFF' is non-zero, a call to one of the routines CTCLDM or CTCLDR will not cause any contour lines to be drawn; instead, the constant-field label will be written. The edge of the grid, the edges of special-value areas, and the edges of out-of-range areas may still be drawn.

Similarly, when 'CFF' is non-zero, a call to the routine CTLBDR will write the constant-field label instead of the labels which would normally be written, and a call to the routine CTLBAM will put the label box for the constant-field label into the area map instead of the label boxes for the normal set of labels.

Workspace Management

Many of the routines in CONPACKT require one or more workspace arrays, some of type REAL and some of type INTEGER. Some routines require more workspace under some conditions than under other conditions. (For example, when label positions are being chosen using either the regular scheme or the penalty scheme, space is required in both the real and integer workspaces and the amount required is larger for a complicated contour plot than it is for a simple one.) Building internal workspaces into a package causes problems; to enlarge such a workspace, the source code of the package must be modified, which is unacceptable. Providing each routine with arguments specifying all of the separate workspaces that it requires leads to complicated and error-prone code.

The workspace management scheme used in CONPACKT is as follows: The user defines one workspace array of type REAL and another of type INTEGER. In the call to CTMESH that initializes the drawing of a contour plot, these arrays appear as arguments (called RWRK and IWRK), together with arguments specifying their lengths (LRWK and LIWK). In subsequent calls to other CONPACKT routines which require workspaces, the same arrays appear as arguments, but the lengths do not. The CONPACKT routines cooperate in using these arrays in such a way as not to interfere with one another. Dynamic enlargement of one workspace at the expense of another becomes possible and the probability of running out of space is reduced.

In general, it is safest not to use the workspace arrays for other purposes between one call to a CONPACKT routine and the next (unless the next is to the routine CTMESH, which initializes the workspace pointers). At the moment, there is only one case in which the contents of the arrays are assumed to be preserved intact: If labels are being positioned using either the "regular" scheme or the "penalty" scheme, the list of label positions is created in the workspace arrays when it is first required and is assumed untouched thereafter. Other cases may arise as a result of further development of the package, however.

It is possible to find out how much space has been used in each of the workspace arrays. The parameters 'IWU' and 'RWU' are zeroed by a call to CTMESH and are updated thereafter to reflect maximum usage of space in the arrays. Thus, one might give the arrays large dimensions, create a typical contour plot, retrieve the values of 'IWU' and 'RWU' to see how much space was actually used, and then reduce the dimensions to more reasonable values.

Workspace usage by some routines cannot be predicted exactly. Upper bounds can be computed, but they may be rather large. For this reason, it may not be possible to absolutely ensure that enough workspace will be available for a given call. Therefore, there is a parameter, called 'WSO', which says what to do when a workspace overflow occurs. The four possibilities are as follows: to terminate after printing an error message, to continue running after printing an error message (this is the default), to just continue running without printing anything, or to do a recoverable-error call to SETER and then continue running without printing anything. Of course, in the latter two cases, incomplete plots may result. It is possible to find out whether or not a workspace overflow has occurred during a given call to a CONPACKT routine; this is done by retrieving the values of the parameters 'IWU' and 'RWU' and comparing them with the dimensions of the workspace arrays IWRK and RWRK.

The following information may be of value in attempting to estimate how much real and integer workspace will be required by each of the user-callable routines of CONPACKT. First, define the following quantities:

Then:

Routines not mentioned above use no workspace.

The Character-Width Multiplier

The parameter 'CWM' is used as a multiplier for all character widths and similar quantities. This makes it possible to scale all such quantities up and down simultaneously. The default value of 'CWM' is 1.

Searching for Highs and Lows

A point of the triangular mesh is defined to be the position of a high if and only if it is in the interior of the mesh and the data value there is greater than the data value at any other point of the mesh within a distance specified by the value of the internal parameter 'HLR'.

A similar definition is used for the position of a low.

Extended High/Low Search Algorithm

It is sometimes the case, in a data field being contoured (particularly if it has been packed and unpacked in such a way as to discretize the distribution of possible values in it), that the same value occurs at more than one mesh point in a small connected region A. If that value is greater than or equal to all the values in some extended region B around A, then it seems reasonable to place a high there, even though none of the mesh points in A satisfies the definition given above. Lows of a similar nature may also exist.

In January, 2002, CONPACK was modified to search for such highs and lows, but only if the user had set the value of the internal parameter 'HLE' non-zero. Such a feature was added to CONPACKT in July, 2004.

If the user has set the value of the internal parameter 'HLE' (which see) non-zero, an additional search will be performed, looking for connected regions in which the field value is constant. Each such region will then be tested to see if it ought to be considered a high or a low (or neither).

Setting 'HLE' equal to 1 will allow each small connected region A to be of any size. If 'HLE' is set larger than 1, it will set an upper limit on the size of each such region; for example, if 'HLE' = 4, then any such region containing more than 4 mesh points will be ignored.

The region B within which the field values must be smaller than or equal to (for a high) or greater than or equal to (for a low) the value at the mesh points of A will be just the union of the neighborhoods defined by the value of 'HLR' for all of the mesh points in A.

The algorithm to do this seems to be only moderately expensive to run, but its timing does depend on the nature of the user's contour field. The feature should probably not be used for fields having very large areas filled with a relative high or low. One problem arises that did not arise for CONPACK: if the high or low is spread out over a relatively large portion of the mesh where the mesh has a small radius of curvature, then the arithmetic mean of the X, Y, and Z coordinates of the points involved can yield a position for the high or low which is significantly off-mesh.

Color-Setting Philosophy

A number of the CONPACKT parameters specify the color of some object (like the informational label) or class of objects (like all contour lines for a given contour level). The default value of each such parameter is -1, which says that the color is to be determined by the current value of one of the GKS color indices. (The polyline color index is used for lines, the text color index for labels, and the fill area color index for filling label boxes.) If the value of the CONPACKT color-setting parameter for a given object is given a value greater than or equal to zero, it specifies the color index of the color in which the object is to be drawn. Before any object is drawn, the values of the GKS color indices affected are saved; after the object is drawn, the saved values are restored.

This structure allows the use of a tiered approach to color setting. If no color setting whatsoever is done, contour plots are drawn entirely in the colors specified by the applicable default values of the GKS color indices. If, on the other hand, prior to calling CONPACKT, one defines the color index "IC" (see the next section, "GKS Considerations") and then uses

      CALL GSPLCI (IC)
to change the GKS polyline color index, then all polylines drawn by CONPACKT change color. Similarly, one may use the statement

      CALL GSTXCI (IC)
to change the GKS text color index and the statement

    CALL GSFACI (IC)
to change the GKS fill area color index; the first will cause labels drawn by CONPACKT to change color and the second will cause label boxes filled by CONPACKT to change color.

If, in addition or instead, CONPACKT color-setting parameters are given values greater than or equal to zero, the objects or classes of objects to which those parameters apply are colored accordingly; these colors are used in preference to values preset by calls to GSPLCI, GSTXCI, or GSFACI.

A final opportunity to set color is provided by the user-supplied versions of the "change" routines, with names of the form CTCHxx; calls to GSPLCI, GSTXCI, and GSFACI may occur in such a routine and take precedence over color setting by any other means. Note that, if color is being set for drawing a label, then either or both of the polyline color index and the text color index may need to be set, depending on whether the labels are being drawn by calls to the GKS routine GPL (to draw polylines stroking out the characters) or by calls to the GKS routine GTX (to draw text). In particular, the routine PLCHHQ, in the package PLOTCHAR, which is called by CONPACKT to draw labels, may be directed by the user to draw high-quality characters, which are stroked, medium-quality characters, which are also stroked, or low-quality characters, which are drawn by GTX.

GKS Considerations

Certain assumptions are made by CONPACKT about the state of GKS, as follows:

(1) Like all the utilities in the NCAR graphics package, CONPACKT assumes that GKS has been opened and that the desired workstations have been opened and activated. The statement

      CALL OPNGKS
calls the SPPS routine OPNGKS, the GKS equivalent of which is

      CALL GOPKS (6,0)
      CALL GOPWK (1,2,1)
      CALL GACWK (1)
creating a single metacode workstation associated with FORTRAN unit 2.

Similarly, at the end of one's program, the workstations must be deactivated and closed and then GKS must be closed. The statement

    CALL CLSGKS
calls the SPPS routine CLSGKS, the GKS equivalent of which is

      CALL GDAWK (1)
      CALL GCLWK (1)
      CALL GCLKS
(2) It is assumed that the aspect source flags for various quantities are set to "individual". (The NCAR GKS package does this by default, but other packages may not.) To make sure that all the aspect source flags are set correctly, use the following code:

      DIMENSION IASF(13)
      ...
      DATA IASF / 13*1 /
      ...
      CALL GSASF (IASF)
(3) Color fill of label boxes is done by CONPACKT using calls to the GKS routine GFA; color fill of contour bands by the user will be done using similar calls. To get solid fill, rather than hollow fill, one must call a GKS routine to set the "fill area interior style":

      CALL GSFAIS (1)
(This is because the default "fill area interior style", as mandated by the GKS standard, is "hollow", rather than "solid".)

(4) Color-setting by CONPACKT is done by executing calls to the GKS routines GSPLCI, GSTXCI, and GSFACI, with user-defined color indices as arguments. The association of these color indices with colors on the workstations must have been defined previously by the user. This should be done by calling the GKS routine GSCR. The statement

      CALL GSCR (IW,IC,RC,GC,BC)
defines, for workstation IW, color index IC, with RGB components RC, GC, and BC. To be consistent with the SPPS routines OPNGKS and CLSGKS, use IW = 1. The value of IC may be any non-negative integer. By default, color index 0 is associated with the color black, which is defined by (RC,GC,BC) = (0.,0.,0.) and is used as the background color, while color index 1 is associated with the color white, which is defined by (RC,GC,BC) = (1.,1.,1.).

SUBROUTINES

All of the CONPACKT routines have six-character names beginning with the letters 'CT'. The user-callable ones are described in detail below.

CTBACK (RPNT,IEDG,ITRI,RWRK,IWRK)

This routine draws a background for a contour plot.

The initial version of CTBACK does very little. User feedback will be useful in determining what this routine is eventually made to do.

Usage

The routine CTBACK may be called at any time after the initialization call to CTMESH. All it does is draw the perimeter of the current viewport; it does this by calling PERIM, in the package GRIDAL.

Arguments

All five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CTCHCF (IFLG)

This routine provides user control as a constant-field message is drawn.

Usage

The routine CTCHCF is not to be called by the user. It is called several times by CONPACKT while a constant-field label is being drawn. The default version of CTCHCF does nothing. A user-supplied replacement may change attributes such as color and line width (by calling the SPPS routine SETUSV or the appropriate GKS routines).

The text of the label being written may be retrieved by means of a "CALL CTGETC ('CTM',CVAL)". The text of the label may be changed by means of a "CALL CTSETC ('CTM',CVAL)"; this should only be done during a call with IFLG = 1 or 3 and, if it is done for one of those two values, it should also be done for the other.

When CTCHCF is called, the parameter 'DVA' will have been set to the value of the field; its value may be retrieved and used by CTCHCF.

Arguments

IFLG (INTEGER, input) is positive if an action is about to be taken, negative if an action has just been completed. The action in question is defined by the absolute value of IFLG, as follows:

CTCHCL (IFLG)

This routine provides user control as contour lines are drawn.

Usage

The routine CTCHCL is not to be called by the user. It is called by the CONPACKT routines CTCLDM and CTCLDR just before and just after the contour lines at each level are drawn. The default version of CTCHCL does nothing. A user-supplied replacement may change attributes such as color and line width (by calling the SPPS routine SETUSV or the appropriate GKS routines).

If the element of the parameter array 'CLU' corresponding to 'PAI' = -1 has been set non-zero to request the drawing of the edge of the grid, then CTCHCL will be called before and after that is done. Similarly, if the element of 'CLU' corresponding to 'PAI' = -2 has been set non-zero, then CTCHCL will be called before and after the drawing of the edges of the out-of-range areas.

When CTCHCL is called, the parameter 'PAI' will have been set to the index of the appropriate contour level (between 1 and 'NCL') or to one of the values -1 or -2. By retrieving the value of 'PAI', CTCHCL can find out what line is being drawn; also, a CTGETx call to retrieve an element of a parameter array like 'CLD' will automatically get the correct one for the line being drawn.

Arguments

IFLG (INTEGER, input) is +1 if a line is about to be drawn, -1 if a line has just been drawn.

CTCHHL (IFLG)

This routine provides user control as high and low labels are drawn.

Usage

The routine CTCHHL is not to be called by the user. It is called several times by CTLBAM and/or CTLBDR while each high or low label is positioned and drawn. The default version of CTCHHL does nothing. A user-supplied replacement may change attributes such as color and line width (by calling the SPPS routine SETUSV or the appropriate GKS routines).

The text of the label may be retrieved by means of a "CALL CTGETC ('CTM',CVAL)". The text of the label may be changed by means of a "CALL CTSETC ('CTM',CVAL)"; this should only be done during a call with IFLG = 1, 3, 5, or 7; if it is done for one of the two values 1 and 3, it should also be done for the other; if it is done for one of the two values 5 and 7, it should also be done for the other.

When CTCHHL is called, the parameter 'DVA' will have been set to the value of the high or low being labelled; its value may be retrieved and used by CTCHHL. Also, the internal parameters 'LBX' and 'LBY' will have been set to the X and Y coordinates of the center point of the label, in the current user coordinate system.

Arguments

IFLG (INTEGER, input) is positive if an action is about to be taken, negative if an action has just been completed. The action in question is defined by the absolute value of IFLG, as follows:

CTCHIL (IFLG)

This routine provides user control as the informational label is drawn.

Usage

The routine CTCHIL is not to be called by the user. It is called several times by CTLBAM and/or CTLBDR while the informational label is positioned and drawn. The default version of CTCHIL does nothing. A user-supplied replacement may change attributes such as color and line width (by calling the SPPS routine SETUSV or the appropriate GKS routines).

The text of the label may be retrieved by means of a "CALL CTGETC ('CTM',CVAL)". The text of the label may be changed by means of a "CALL CTSETC ('CTM',CVAL)"; this should only be done during a call with IFLG = 1 or IFLG = 3, and, if it is done for one of those values, it should be done for the other.

The internal parameters 'LBX' and 'LBY' will have been set to the X and Y coordinates of the center point of the label, in the current user coordinate system.

Arguments

IFLG (INTEGER, input) is positive if an action is about to be taken, negative if an action has just been completed. The action in question is defined by the absolute value of IFLG, as follows:

CTCHLL (IFLG)

This routine provides user control as contour line labels are drawn.

Usage

The routine CTCHLL is not to be called by the user. It is called several times by CTLBAM and/or CTLBDR while each contour-line label is positioned and drawn. The default version of CTCHLL does nothing. A user-supplied replacement may change attributes such as color and line width (by calling the SPPS routine SETUSV or the appropriate GKS routines).

The text of the label may be retrieved by means of a "CALL CTGETC ('CTM',CVAL)". The text of the label may be changed by means of a "CALL CTSETC ('CTM',CVAL)"; this should only be done during a call with IFLG = 1 or 3 and, if it is done for one of those values, it should be done for the other.

When CTCHLL is called, the parameter 'PAI' will have been set to the index of the appropriate contour level. Thus, parameters associated with that level may easily be retrieved by calls to CTGETx. Also, the parameter 'DVA' will have been set to the contour level value and the internal parameters 'LBX' and 'LBY' will have been set to the X and Y coordinates of the center point of the label, in the current user coordinate system.

Arguments

IFLG (INTEGER, input) is positive if an action is about to be taken, negative if an action has just been completed. The action in question is defined by the absolute value of IFLG, as follows:

CTCICA (RPNT,IEDG,ITRI,RWRK,IWRK, ... )

(The remaining arguments are ICRA,ICA1,ICAM, ICAN,XCPF, YCPF, XCQF, and YCQF.)

This routine creates a GKS cell array, using color indices determined by examining where the user's contours lie relative to the cell array. It ignores all triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle. If the 3D package TDPACK is being used to project the triangular mesh into the plane, then the routine CTTDCA should probably be called instead, since it can be made to create a much better picture of the mesh than CTCICA can do.

Usage

The routine CTCICA may be called at any time after the initialization call to CTMESH. It is given arrays defining a triangular mesh of data, a real workspace array, an integer workspace array, an array in which a GKS cell array is to be created, and dimensioning and positioning information for the cell array. It creates the cell array by associating with each cell of it an area identifier (let's call it IAID) and then using IAID to generate a color index to store in that cell; there are three possible values of IAID:

Note that, to be sure of distinguishing all three possibilities from each other, it is necessary to give elements -1 and -2 of the parameter array 'AIA' values which are different from the value of any other element of 'AIA' or 'AIB'.

Note also that the "off-grid" value is used in cases in which it would not have been by the CONPACK routine CPCICA; in particular, it may be used for points inside mapped triangles of the triangular mesh having one or two vertices that map out-of-range.

Once an area identifier has been associated with a particular cell, that area identifier must be mapped into a color index to be stored as the value of the corresponding element of the cell array. The internal parameter 'CAF' determines how area identifiers are mapped into color indices, as follows:

The default value of 'CAF' is zero, so that CTSCAE is not called: if IAID is non-negative, it is used as the desired color index for a particular cell; otherwise, a zero is used.

Note that the routine CTCICA works differently from the routine CPCICA, in CONPACK, which allowed one to modify some elements of an existing cell array, while leaving others, in out-of-range areas or off-grid areas or special-value areas, unchanged. CPCICA could do that because the CONPACK routine CPMPXY implemented full inverse mappings, which the CONPACKT routine CTMXYZ does not do.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

ICRA (INTEGER array, dimensioned ICA1 by "n", where "n" is greater than or equal to the value of the argument ICAN, input/output) is the user's cell array.

ICA1 (INTEGER, input) is the first dimension of the FORTRAN array ICRA, which contains the user's cell array.

ICAM (INTEGER, input) is the first dimension of the user's cell array.

ICAN (INTEGER, input) is the second dimension of the user's cell array.

XCPF and YCPF (REAL, input) are the coordinates, in the fractional coordinate system, of a point P, which is the point at that corner of the rectangular area into which the cell array maps that is also a corner of the cell with indices (1,1).

XCQF and YCQF (REAL, input) are the coordinates, in the fractional coordinate system, of a point Q, which is the point at that corner of the rectangular area into which the cell array maps that is also a corner of the cell with indices (ICAM,ICAN).

CTCLAM (RPNT,IEDG,ITRI,RWRK,IWRK,IAMA)

This routine adds contour lines to an area map as part of the process of drawing a solid-fill contour plot. It ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTCLAM may be called at any time after the initialization call to CTMESH to add contour lines generated from the data defined on the triangular mesh to the area map in the array IAMA. The area map must previously have been initialized by a call to the routine ARINAM, in the package AREAS.

The contour lines added to the area map are as specified by the first 'NCL' elements of the parameter arrays 'CLV', 'AIA', and 'AIB'. If 'NCL' is zero, CTPKCL is called to generate these values. The contour levels defined by the first 'NCL' elements of the parameter array 'CLV' are then examined. If a given contour level is associated with a non-zero value of 'AIA' and/or a non-zero value of 'AIB', the contour lines at that contour level are added to the area map. If there is an associated non-zero value of 'AIA', it is used as the area identifier for the area "above" the line (where field values are greater than they are along the line); otherwise, a zero is used. If there is an associated non-zero value of 'AIB', it is used as the area identifier for the area "below" the line (where field values are less than they are along the line); otherwise, a zero is used. Note that a given contour level may occur more than once in the internal parameter array 'CLV', but there must be at most one non-zero value of 'AIA' and at most one non-zero value of 'AIB' associated with it; otherwise, an error exit occurs. If the parameter 'T2D' has a non-zero value, the contour lines are smoothed, using cubic splines under tension.

Other types of lines are also added to the area map by CTCLAM: the edge of the current viewport and possibly a set of vertical lines within the viewport; the edge of the grid; and the edges of out-of-range areas, if any. The area identifier for the outside of the viewport is always -1. Elements of the parameter array 'AIA' for 'PAI' = -1 and -2 may be used to specify the area identifiers to be used for the outside of the grid and the inside of an out-of-range area, respectively; the default values of the first is 0 and the default value of the second is -1. Area identifiers for all other sides of these edges are determined from the area-identifier information given for the contour levels.

The lines added to the area map are put into two edge groups, one with group identifier 'GIC' and another with group identifier 'GIS'. (The edge of the viewport may actually be added twice, once to each group.) The edge group 'GIC' is the more important of the two; it really defines the division of the plane into contour bands, out-of-range areas, and outside-the-grid areas. The edge group 'GIS' only receives the edge of the viewport and a collection of vertical lines; its object is to break up the areas defined by the other edge group into vertical pieces, creating simpler polygons on devices that might not handle more complicated ones. Whether or not edge group 'GIS' is created is under control of the user; for more information, see the descriptions of the parameters 'GIS' and 'NVS'.

Lines are added to the area map in the following order: the edge of the viewport and the vertical lines within it, edges of the out-of-range areas, if any; the edge of the grid; and the contour lines, in order of increasing contour level.

If, during the last call to CTMESH, the data being contoured were found to be essentially constant, then no contour lines are added to the area map; the other lines are added, however.

Arguments

The first five arguments are arrays used in a previous call to the one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

IAMA (INTEGER array, dimensioned as specified in a call to ARINAM, in the package AREAS) is the array containing the area map to which contour lines are to be added.

CTCLDM (RPNT,IEDG,ITRI,RWRK,IWRK,IAMA,RTPL)

This routine draws contour lines masked by an existing area map. The object of this may be simply to avoid drawing contour lines through label boxes, but the routine may be used for more complicated tasks, like limiting the drawing of contour lines to the ocean areas on an EZMAP background. It ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTCLDM may be called at any time after the initialization call to CTMESH to draw contour lines masked by an existing area map. Actually, CTCLDM does not draw the lines; it generates them, masks them against a user-specified area map, and generates a set of calls to a user-specified routine (one call for each polyline resulting from the masking process). Each such polyline lies entirely within precisely one of the areas defined by the area map. The user routine may use the information provided by its arguments, describing the area the polyline is in, to decide whether or not to draw the polyline.

The contour lines generated are those specified by the first 'NCL' elements of the parameter arrays 'CLV' and 'CLU'. If 'NCL' is zero, CTPKCL is called to generate these values. Each element of 'CLV' specifies a contour level and the corresponding element of 'CLU' specifies whether or not contour lines are to be generated at that level. If the parameter 'T2D' has a non-zero value, the contour lines are smoothed, using cubic splines under tension.

If the element of the parameter array 'CLU' corresponding to 'PAI' = -1 is non-zero, the edge of the grid is also generated. If the element of 'CLU' corresponding to 'PAI' = -2 is non-zero, the edges of out-of-range areas (if any) are generated. The default values are such that neither type of edge is generated.

Groups of lines are generated in the following order: contour lines for each of the specified levels, in ascending numeric order; the edges of special-value areas, if any; the edges of out-of-range areas, if any; the edge of the grid.

The color, dash pattern, and line width to be used for the lines drawn may be specified by elements of the parameter arrays 'CLC', 'CLD', and 'CLL', respectively. Each of these contains elements corresponding to values of 'PAI' from 1 to 'NCL' and two special elements, corresponding to 'PAI' = -1 and -2. Before and after each group of lines is generated, the routine CTCHCL is called; a user-supplied version of this routine may override the settings of color, dash pattern, and line width. (Also, of course, the user routine which actually does the drawing may override the settings of these quantities.)

If the dash-pattern-usage parameter 'DPU' is zero, it is assumed that lines are to be drawn by calling the SPPS routine CURVE; specified dash patterns are not used. If 'DPU' is greater than zero, it is assumed that lines are to be drawn by calling the DASHCHAR routine CURVED, while if 'DPU' is less than zero, it is assumed that lines are to be drawn by calling the DASHPACK routine DPCURV; in either case, specified dash patterns are used. If 'DPU' is non-zero and ABS('LLP') = 1, then, for those lines which are to be labelled, the dash pattern is constructed by replicating the dash pattern specified by the appropriate element of 'CLD' 'DPU' times and then adding to that the numeric label specified by the appropriate element of 'LLT'.

If, during the last call to CTMESH, the data being contoured were found to be essentially constant, then no contour lines are generated; instead, the constant-field label is written. Other lines are still generated.

Arguments

The first five arguments are arrays used in a previous call to the one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

IAMA (INTEGER array, dimensioned as specified in a call to ARINAM, in the package AREAS, input/output) is the array containing the area map which is to be used to mask the contour lines as they are drawn.

RTPL (EXTERNAL subroutine) is the user subroutine which is to process the polylines which result from masking the generated contour lines and other edges against the area map. It must be declared EXTERNAL in the routine which calls CTCLDM. It will be called repeatedly and must have the following form:

      SUBROUTINE RTPL (XCS,YCS,NCS,IAI,IAG,NAI)
      DIMENSION XCS(*),YCS(*),IAI(*),IAG(*)
      ...
      (CODE TO PROCESS POLYLINE DEFINED BY ARGUMENTS)
      ...
      RETURN
      END
The real arrays XCS and YCS hold the X and Y coordinates of NCS points defining a polyline which is to be considered for drawing. For each I greater than or equal to 1 and less than or equal to NAI, IAI(I) is the area identifier for the area in which the polyline lies, relative to the edge group IAG(I). The X and Y coordinates are all normalized device coordinates and it may be assumed that the appropriate SET call has been done. If it is decided to draw the line, it may be done with a call to the SPPS routine CURVE, to the DASHCHAR routine CURVED, to the DASHPACK routine DPCURV, or to the GKS routine GPL. The value of 'PAI' will have been set to the appropriate value (1 through 'NCL', -1, or -2) for the line of which the polyline is a part. The color and line width will have been set as implied by the values of the appropriate elements of the parameter arrays 'CLC' and 'CLL'. The dash pattern will have been defined as implied by the value of the appropriate elements of the parameter arrays 'CLD' and 'CLU' and the value of the parameter 'DPU'. If a dash pattern is defined, it may be retrieved by a "CALL CTGETC ('CTM',CVAL)".

If the only object of using CTCLDM is to avoid drawing contour lines through label boxes, then the CONPACKT routine CTDRPL may be used for RTPL. In the routine that calls CTCLDM, insert the declaration

      EXTERNAL CTDRPL
and then use CTDRPL for the last argument.

For more information, see the description of the argument LPR of the subroutine ARDRLN, in the documentation of the package AREAS.

CTCLDR (RPNT,IEDG,ITRI,RWRK,IWRK)

This routine draws contour lines. It ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTCLDR may be called at any time after the initialization call to CTMESH to draw contour lines.

The contour lines drawn are those specified by the first 'NCL' elements of the parameter arrays 'CLV' and 'CLU'. If 'NCL' is zero, CTPKCL is called to generate these values. Each element of 'CLV' specifies a contour level and the corresponding element of 'CLU' specifies whether or not contour lines are to be drawn at that level and whether or not the lines are to be labelled. If the parameter 'T2D' has a non-zero value, the contour lines are smoothed, using cubic splines under tension.

If the element of the parameter array 'CLU' corresponding to 'PAI' = -1 is non-zero, the edge of the grid is also drawn. If the element of 'CLU' corresponding to 'PAI' = -2 is non-zero, the edges of out-of-range areas (if any) are drawn. The default values are such that neither type of edge is drawn.

Groups of lines are drawn in the following order: contour lines for each of the specified levels, in ascending numeric order; the edges of special-value areas, if any; the edges of out-of-range areas, if any; the edge of the grid.

The color, dash pattern, and line width to be used for the lines drawn may be specified by elements of the parameter arrays 'CLC', 'CLD', and 'CLL', respectively. Each of these contains elements corresponding to values of 'PAI' from 1 to 'NCL' and two special elements, corresponding to 'PAI' = -1 and -2. Before and after each group of lines is drawn, the routine CTCHCL is called; a user-supplied version of this routine may override the settings of color, dash pattern, and line width.

If the dash-pattern-usage parameter 'DPU' is zero, lines are drawn by calling the SPPS routine CURVE; they are all solid and unlabelled. If 'DPU' is greater than zero, lines are drawn by calling the DASHCHAR routine CURVED, while if 'DPU' is less than zero, lines are drawn by calling the DASHPACK routine DPCURV; in either case, they are solid or dashed, depending on the dash pattern specified by the appropriate element of 'CLD'. If 'DPU' is non-zero and ABS('LLP') = 1, the dash pattern for those lines which are to be labelled is constructed by replicating the dash pattern specified by the appropriate element of 'CLD' 'DPU' times and then adding to it the characters specified by the apppropriate element of 'LLT'. If 'DPU' is non-zero and ABS('LLP') is greater than 1, the lines drawn will pass through any labels drawn by CTLBDR; if this is undesirable, CTLBAM can be used to put the label boxes into an area map and CTCLDM can be used to draw only those portions of the contour lines which do not lie inside the label boxes.

If, during the last call to CTMESH, the data being contoured were found to be essentially constant, then no contour lines are drawn; instead, the constant-field label is written. Other lines are still drawn.

Arguments

All five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CTCLTR (RPNT,IEDG,ITRI,RWRK,IWRK,CLVL,IJMP, ... )

(The remaining arguments are IRW1, IRW2, and NRWK.)

This routine traces the contour lines at a given level and retrieves them for some sort of user-defined processing. The contour lines are traced in such a way that lower field values (less than CLVL) are to the left and higher field values (greater than CLVL) are to the right (where "left" and "right" are defined from the standpoint of someone standing at one point of the contour line, looking toward the next).

CTCLCR ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTCLTR may be called at any time after the initialization call to CTMESH. It is called using code like the following:

      IJMP=0
  101 CALL CTCLTR (RPNT,IEDG,ITRI,RWRK,IWRK,CLEV,IJMP,IRW1,IRW2,NRWK)
      IF (IJMP.NE.0) THEN
        (PROCESS SEGMENT OF CONTOUR LINE THAT HAS BEEN PUT IN RWRK.)
        GO TO 101
      END IF
CTCLTR is called repeatedly. Initially, IJMP is zeroed. Upon each return from CTCLTR, if the value of IJMP is non-zero, X and Y coordinates have been placed in the real workspace array RWRK and the values of IRW1, IRW2, and NRWK have been set to indicate where the coordinates were put. After the coordinates have been processed, CTCLTR is called again. If, upon return from CTCLTR, the value of IJMP is zero, all contour lines at the specified level have been processed.

If the parameter 'T2D' has a non-zero value, the contour lines are smoothed, using cubic splines under tension.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CLEV (REAL, input) is the desired contour level.

IJMP (INTEGER, input/output) is initially set to zero by the user. Upon return from CTCLTR, it will be non-zero if coordinates of a contour line segment have been placed in RWRK; it will be zero if all contour line segments at the given level have been generated.

IRW1 (INTEGER, output) is a pointer to the place in RWRK where X coordinates have been stored. They will be in RWRK(IRW1+I) for I = 1, 2, ..., NRWK.

IRW2 (INTEGER, output) is a pointer to the place in RWRK where Y coordinates have been stored. They will be in RWRK(IRW2+I) for I = 1, 2, ..., NRWK.

NRWK (INTEGER, output) is the number of points on the contour line segment. The points comprising the segment will be (RWRK(IRW1+I),RWRK(IRW2+I)) for I = 1, 2, ... NRWK.

CTDRPL (XCS,YCS,NCS,IAI,IAG,NAI)

This routine provides a useful polyline-drawer for the routine CTCLDM (the description of which appears earlier in this section).

Usage

If CTCLDM is called, and the only object of using it, instead of CTCLDR, to draw contour lines is to avoid drawing the lines through labels, then, in the routine which calls CTCLDM, put the declaration

      EXTERNAL CTDRPL
and, in the call to CTCLDM, use CTDRPL for the argument RTPL. Each time CTDRPL is called, it draws the polyline defined by its first three arguments if, and only if, none of the area identifiers defined by the other three arguments is negative.

Arguments

XCS (a REAL array of dimension at least NCS, input) is an array containing the X coordinates of NCS points defining a polyline.

YCS (a REAL array of dimension at least NCS, input) is an array containing the Y coordinates of NCS points defining a polyline.

NCS (INTEGER, input) is the number of points defining the polyline.

IAI (an INTEGER array of dimension at least NAI, input) is an array of area identifiers for the area in which the polyline lies. For each I from 1 to NAI, IAI(I) is the area identifier of the area with respect to the edge group IAG(I).

IAG (an INTEGER array of dimension at least NAI, input) is an array of group identifiers. See the description of IAI, above.

NAI (INTEGER, input) is the number of area identifiers in the array IAI and the number of group identifiers in the array IAG.

CTGETC (PNAM,CVAL)

This routine is used to get the current value of a parameter of type CHARACTER.

Usage

Use the statement 

      CALL CTGETC (PNAM,CVAL)
at any time to retrieve in CVAL the current character value of the parameter whose name is PNAM. If that parameter is an array, the array element specified by the current value of the parameter 'PAI' will be the one retrieved.

Arguments

PNAM (CHARACTER, input) is the name of a parameter whose character value is to be retrieved. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of just 'LLT', use 'LLT - LINE LABEL TEXT'.

CVAL (CHARACTER, output) is a variable in which the value of the parameter specified by PNAM is to be returned.

CTGETI (PNAM,IVAL)

This routine is used to get the current integer value of a parameter.

Usage

Use the statement 

      CALL CTGETI (PNAM,IVAL)
at any time to retrieve in IVAL the current integer value of the parameter whose name is PNAM. If that parameter is an array, the array element specified by the current value of the parameter 'PAI' will be the one retrieved.

Arguments

PNAM (CHARACTER, input) is the name of a parameter whose integer value is to be retrieved. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of just 'LLP', use 'LLP - LINE LABEL POSITIONING'.

IVAL (INTEGER, output) is a variable in which the value of the parameter specified by PNAM is to be returned. If the internal parameter is of type INTEGER and has the value "i", then IVAL = i; if the internal parameter is of type REAL and has the value "r", then IVAL = INT(r).

CTGETR (PNAM,RVAL)

This routine is used to get the current real value of a parameter.

Usage

Use the statement 

      CALL CTGETR (PNAM,RVAL)
at any time to retrieve in RVAL the current real value of the parameter whose name is PNAM. If that parameter is an array, the array element specified by the current value of the parameter 'PAI' will be the one retrieved.

Arguments

PNAM (CHARACTER, input) is the name of a parameter whose real value is to be retrieved. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of just 'SFS', use 'SFS - SCALE FACTOR SELECTOR'.

RVAL (REAL, output) is a variable in which the value of the parameter specified by PNAM is to be returned. If the internal parameter is of type INTEGER and has the value "i", then RVAL = REAL(i); if the internal parameter is of type REAL and has the value "r", then RVAL = r.

CTLBAM (RPNT,IEDG,ITRI,RWRK,IWRK,IAMA)

This routine is used to add label boxes (for the informational label, high and low labels, and contour-line labels) to an area map. The ultimate object of this will usually be to prevent contour lines drawn by CTCLDM from passing through labels or to prevent fill of the label boxes as contour bands are filled.

CTLBAM ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTLBAM may be called at any time after the initialization call to CTMESH to add label boxes to an area map. If, during the last call to CTMESH, the data being contoured were found to be essentially constant, then the box for the constant-field label is added to the area map. Otherwise, boxes for the informational label, the high/low labels, and/or the contour-line labels are added.

A box for the informational label is added only if the parameter 'ILT', which specifies the text of that label, is non-blank.

Boxes for the high labels are added only if the parameter 'HIT', which specifies the text of those labels, is non-blank.

Boxes for the low labels are added only if the parameter 'LOT', which specifies the text of those labels, is non-blank.

Boxes for the contour line labels are added only if the parameter 'LLP', which says how those labels are to be positioned, has an absolute value of 2 or 3, and if, for some I between 1 and 'NCL', inclusive, the Ith element of the parameter array 'CLU' has a value implying that contour lines at the contour level specified by the Ith element of 'CLV' are to be labelled.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

IAMA (INTEGER array, dimensioned as specified in a call to ARINAM) is the array holding the area map to which the label boxes are to be added.

CTLBDR (RPNT,IEDG,ITRI,RWRK,IWRK)

This routine draws labels (an informational label, high and low labels, and line labels). It ignores triangles of the triangular mesh that are blocked, as specified by the current values of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTLBDR may be called at any time after the initialization call to CTMESH to draw labels. If, during the last call to CTMESH, the data being contoured were found to be essentially constant, then the constant-field label is drawn. Otherwise, the informational label, the high/low labels, and/or the contour-line labels are drawn.

The informational label is drawn only if the parameter 'ILT', which specifies the text of that label, is non-blank.

High labels are drawn only if the parameter 'HIT', which specifies the text of those labels, is non-blank.

Low labels are drawn only if the parameter 'LOT', which specifies the text of those labels, is non-blank.

Contour line labels are drawn only if the parameter 'LLP', which says how those labels are to be positioned, has an absolute value of 2 or 3, and if, for some I between 1 and 'NCL', inclusive, the Ith element of the parameter array 'CLU' has a value implying that contour lines at the contour level specified by the Ith element of 'CLV' are to be labelled.

Arguments

All five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CTMESH (RPNT,NPNT,LOPN,IEDG,NEDG,LOEN, ... )

(The remaining arguments are ITRI, NTRI, LOTN, RWRK, LRWK, IWRK, and LIWK.)

Initializes the contouring of a triangular array of data. CTMESH ignores triangles of the triangular mesh that are blocked by the user, as specified by the low-order bits of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle.

Usage

The routine CTMESH is called to initialize the process of drawing a contour plot from a triangular array of data. The arguments define the data arrays, a real workspace array, and an integer workspace array. The dimensions of all the arrays are transferred to variables in COMMON, so that, in calls to other CONPACKT routines, those dimensions may be omitted. The internal pointers which are used to manage workspace use are initialized. It is decided what the ranges of X and Y coordinates used to draw contour lines and position labels ought to be. If CONPACKT is to call SET, appropriate arguments are determined and SET is called; otherwise, GETSET is called to retrieve the arguments from the user's call to SET. The list of label positions (if any) left over from previous calls to the package is discarded. If contour levels are to be chosen by the package, 'NCL' is zeroed so that the levels will be chosen when required. The minimum and maximum values in the data array are located and it is decided whether the data are essentially constant. Numeric-label parameters which depend on the range of values in the data array are initialized. A scale factor may be chosen.

Arguments

RPNT (REAL array, dimensioned greater than or equal to NPNT x LOPN, input) is the user's mesh-point array.

NPNT (INTEGER, input) is the number of elements in RPNT (that is, the number of points in the triangular mesh, times LOPN).

LOPN (INTEGER, input) is the length of a point node.

IEDG (INTEGER array, dimensioned greater than or equal to NEDG x LOEN, input) is the user's edge array.

NEDG (INTEGER, input) is the number of elements in IEDG (that is, the number of edges of the triangular mesh, times LOEN).

LOEN (INTEGER, input) is the length of an edge node.

ITRI (INTEGER array, dimensioned greater than or equal to NTRI x LOTN, input) is the user's triangle array.

NTRI (INTEGER, input) is the number of elements in ITRI (that is, the number of triangles of the triangular mesh, times LOTN).

LOTN (INTEGER, input) is the length of a triangle node.

RWRK (REAL array, dimensioned LRWK, input/output) is the real work array.

LRWK (INTEGER, input) is the length of RWRK.

IWRK (INTEGER array, dimensioned LIWK, input/output) is the integer work array.

LIWK (INTEGER, input) is the length of IWRK.

CTMXYZ (IMAP,XINP,YINP,ZINP,XOTP,YOTP)

This routine defines mappings from the 3D coordinate system of the triangular mesh to the 2D "user" coordinate system in which the mapped contours are being drawn.

Usage

CTMXYZ is not to be called by the user. It is called by various CONPACKT routines when the parameter 'MAP' is non-zero. A user may supply his or her own version of the routine in order to define other mappings.

The first argument in a call to CTMXYZ will be positive if the call is intended to map the X, Y, and Z coordinates of a point from the 3D space in which the triangular mesh is defined to X and Y "user" coordinates in the 2D space of the drawing plane.

The first argument in a call to CTMXYZ will be negative only if the parameter 'ORV' has been given a non-zero value, in which case the call is meant to determine whether or not a point in the 2D space of the drawing plane is "out-of-range". "Out-of-range" may mean "not a part of the mapped image of the triangular mesh", but it is more likely to mean "not a part of the mapped image of the surface (for example, the globe) on which the triangular mesh lies".

The default version of CTMXYZ is as follows:

      SUBROUTINE CTMXYZ (IMAP,XINP,YINP,ZINP,XOTP,YOTP)
        DATA RTOD / 57.2957795130823 /  !  (radians to degrees)
        IF (IMAP.EQ.1) THEN
          RLAT=RTOD*ASIN(ZINP/SQRT(XINP*XINP+YINP*YINP+ZINP*ZINP))
          IF (XINP.EQ.0..AND.YINP.EQ.0.)
            RLON=0.
          ELSE
            RLON=RTOD*ATAN2(YINP,XINP)
          END IF
          CALL MAPTRA (RLAT,RLON,XOTP,YOTP)
        ELSE IF (IMAP.EQ.-1) THEN
          CALL MAPTRI (XINP,YINP,XOTP,YOTP)
        ELSE IF (IMAP.EQ.2) THEN
          CALL TDPRPT (XINP,YINP,ZINP,XOTP,YOTP)
        ELSE
          XOTP=XINP
          YOTP=YINP
        END IF
        RETURN
      END
When CTMXYZ is called with IMAP = 1, the incoming X, Y and Z coordinates are assumed to represent points on the surface of a sphere of radius 1; from these, it computes values of latitude and longitude and calls the EZMAP routine MAPTRA to find the X and Y coordinates on the map of the projection of the specified point on the globe; those coordinates are returned as the outgoing X and Y coordinates.

When IMAP = -1, the incoming X and Y coordinates are assumed to be the X and Y coordinates of a projected point on the map; the EZMAP routine MAPTRI is called to find the latitude and longitude of the original point on the globe and those values are returned as the outgoing X and Y coordinates. If the point is off the map (as, for example, when an orthographic projection is used and the point is outside the circle of radius 1 into which the visible hemisphere of the earth is mapped by that projection), then MAPTRI will return the value 1.E12 (which should be declared the "out-of-range" value for CONPACKT).

When CTMXYZ is called with IMAP = 2, the incoming X, Y, and Z coordinates are assumed to represent points on an arbitrary mesh in 3-space; these are projected to an image plane by calling the routine TDPRPT, in the 3D package TDPACK.

Note that calling CTMXYZ with IMAP = -2 does not use TDPACK; furthermore, when 'MAP' is given the value 2, 'ORV' should not be set non-zero; there is no "out-of-range" value.

If IMAP is anything else, the input X and Y coordinates are simply returned as the output X and Y coordinates.

Note that CTMXYZ is never called with IMAP = 0 (as was the case for the analogous CONPACK routine CPMPXY).

If CTMXYZ is changed to do a new mapping for a particular value of 'MAP', it must be made to respond properly to a first argument with value +'MAP' or -'MAP'.

Arguments

IMAP (INTEGER, input) is greater than zero if the object of the call is to do a forward mapping and less than zero if the object of the call is to check the "out-of-range" status of a point in the user coordinate system. The absolute value of IMAP will be equal to the current value of the parameter 'MAP', identifying the mapping to be used.

XINP (REAL, input) is used in one of two ways:

YINP (REAL, input) is used in one of two ways:

ZINP (REAL, input) is only used when IMAP is greater than zero, in which case it is the Z coordinate of a point on the triangular mesh. When IMAP is less than zero, ZINP is ignored.

XOTP (REAL, output) and YOTP (REAL, output) are used in one of two ways:

To reiterate: if the point (XINP,YINP) cannot be mapped for any reason, some recognizable impossible value should be returned for both of XOTP and YOTP and the internal parameter 'ORV' should be given that value, thereby allowing CONPACKT routines that call CTMXYZ to determine whether or not a point being mapped is visible or not. The value used for this purpose by the EZMAP routines MAPTRA and MAPTRI is 1.E12.

CTMVIW (IWKO,IWKN,LWKN)

This routine is called to move what CONPACKT has in the current integer workspace array to a new array.

Usage

When, in the execution of a CONPACKT routine, the amount of space left in the integer workspace array is found to be insufficient, if the internal parameter 'WSO' has the value 3, the error-handling routine SETER is called with an appropriate error message. If, in addition, the user has turned recovery mode on, execution continues and, eventually, control is returned to the user. At that point, the user should detect the fact that an error has occurred. If he/she chooses to try to recover from the error, CTMVIW may be of use: it may be called to move everything from the current integer workspace array to a new (and presumably bigger) one, after which it may be possible to resume execution.

Arguments

IWKO (an input array of type INTEGER, dimensioned as specified in a previous call to CTMESH or CTMVIW) is the current ("old") integer workspace array.

IWKN (an output array of type INTEGER, dimensioned LWKN) is the array which is to become the new integer workspace array.

LWKN (INTEGER, input) is the dimension of the array IWKN.

CTMVRW (RWKO,RWKN,LWKN)

This routine is called to move what CONPACKT has in the current real workspace array to a new array.

Usage

When, in the execution of a CONPACKT routine, the amount of space left in the real workspace array is found to be insufficient, if the internal parameter 'WSO' has the value 3, the error-handling routine SETER is called with an appropriate error message. If, in addition, the user has turned recovery mode on, execution continues and, eventually, control is returned to the user. At that point, the user should detect the fact that an error has occurred. If he/she chooses to try to recover from the error, CTMVRW may be of use: it may be called to move everything from the current real workspace array to a new (and presumably bigger) one, after which it may be possible to resume execution.

Arguments

RWKO (an input array of type REAL, dimensioned as specified in a previous call to CTMESH or CTMVRW) is the current ("old") real workspace array.

RWKN (an output array of type REAL, dimensioned LWKN) is the array which is to become the new real workspace array.

LWKN (INTEGER, input) is the dimension of the array RWKN.

CTPKCL (RPNT,IEDG,ITRI,RWRK,IWRK)

This routine is called to pick a set of contour levels.

Usage

Normally, CTPKCL is called by CONPACKT when the contour levels are needed; it may be called by the user (after the initialization call to CTMESH) when it is desired to modify the resulting parameter arrays specifying contour levels and associated quantities in some way.

If the contour-level-selection parameter 'CLS' is zero, CTPKCL does nothing. If the constant-field-flag 'CFF' is non-zero, indicating that, on the last call to CTMESH, the data were found to be essentially constant, CTPKCL does nothing.

If neither of the conditions mentioned in the last paragraph arises, contour levels are picked. For a complete description of the various possible ways in which this might be done, see the description of the parameter 'CLS'. In any case, 'NCL' is set to the number of contour levels picked and elements 1 through 'NCL' of the parameter array 'CLV' are set to the levels themselves. The Ith elements of the associated parameter arrays are set as follows:

      'CLU' = 1 OR 3              CONTOUR LEVEL USE FLAG
      'AIA' = I+1                 AREA IDENTIFIER ABOVE THE LEVEL
      'AIB' = I                   AREA IDENTIFIER BELOW THE LEVEL
      'LLT' = ' '                 CONTOUR LINE LABEL TEXT
      'CLD' = '$$$$$$$$$$$$$$$$'  CONTOUR LINE DASH PATTERN
      'CLC' = -1                  CONTOUR LINE COLOR
      'LLC' = -1                  CONTOUR LINE LABEL COLOR
      'CLL' = -1.                 CONTOUR LINE LINE WIDTH
Thus, after CTPKCL is called, the situation for each contour level is this: Contour lines at the level are to be generated by calls to CTCLDM or CTLBDR; the lines will be labelled if 'CLU' = 3, but not if 'CLU' = 1. If CTCLAM is called, the lines will be added to the area map; the identifier used for areas "above" the lines will be the contour level index plus one and the identifier used for areas "below" the lines will be the contour level index. The text of the label associated with the level is, as yet, unspecified (see the description of the subroutine CTPKLB, below). The dash pattern for the level is solid, and neither the color of the line, the color of the labels on it, nor its width are to be set. By resetting elements in these parameter arrays, the user can change the situation in various ways.

Arguments

All five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CTPKLB (RPNT,IEDG,ITRI,RWRK,IWRK)

This routine is called to pick a set of labels for labelled contour levels.

Usage

Normally, CTPKLB is called by CONPACKT when labels for the contour levels are needed; it may be called by the user (after the initialization call to CTMESH) when it is desired to modify the resulting parameter arrays specifying the labels.

If the constant-field-flag 'CFF' is non-zero, indicating that, on the last call to CTMESH, the data were found to be essentially constant, CTPKLB does nothing; otherwise, it examines the first 'NCL' elements of the parameter array 'CLV', which defines the contour levels, and the associated parameter arrays, looking for levels which are to be labelled ('CLU' = 2 or 3) for which no label is specified ('LLT' = ' ', a single blank). If any such levels are found, labels are generated for them.

The scale factor 'SFU' may be set as a byproduct of choosing the labels. See the description of the parameter 'SFS'.

After calling CTPKLB, a user program may examine the generated labels and change them in various ways.

Arguments

All five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

CTRSET

Resets all parameters to their initial default values.

Usage

Use "CALL CTRSET" to reset all parameters to their default values.

Arguments

None.

CTSCAE (ICRA,ICA1,ICAM,ICAN, ... )

(The remaining arguments are XCPF, YCPF, XCQF, YCQF, IND1, IND2, ICAF, and IAID.)

Updates a particular element of a user's cell array.

Usage

CTSCAE is not called by the user. When a user calls the routine CTCICA and the internal parameter 'CAF' is negative, CTSCAE is called by CTCICA exactly once for each cell in the cell array. The default version of CTSCAE just sets the cell's color index equal to the area identifier associated with the cell, if that area identifier is greater than or equal to zero; otherwise, it does nothing. A user-supplied version of CTSCAE may do something more complicated; for example, it may take into account both the value of the area identifier associated with the cell and the current value of the color index for the cell in determining the new value of the color index for the cell.

Arguments

The first eight arguments of CTSCAE describe the user's cell array and are taken directly from the user's call to CTCICA:

ICRA (INTEGER array, dimensioned ICA1 by "n", where "n" is greater than or equal to the value of the argument ICAN, input/output) is the user's cell array. Generally speaking, a user version of CTSCAE should only reset the value of the single element ICRA(IND1,IND2).

ICA1 (INTEGER, input) is the first dimension of the FORTRAN array ICRA, which contains the user's cell array.

ICAM (INTEGER, input) is the first dimension of the user's cell array.

ICAN (INTEGER, input) is the second dimension of the user's cell array.

XCPF and YCPF (REAL, input) are the coordinates, in the fractional coordinate system, of a point P. P is the point at that corner of the rectangular area into which the cell array maps that corresponds to the cell (1,1).

XCQF and YCQF (REAL, input) are the coordinates, in the fractional coordinate system, of a point Q. Q is the point at that corner of the rectangular area into which the cell array maps that corresponds to the cell (ICAM,ICAN).

IND1 and IND2 (INTEGER, input) are the indices of an element in the cell array that is to be reset.

ICAF (INTEGER, input) is the value of the internal parameter 'CAF', which will be a negative integer (because, when 'CAF' is greater than or equal to zero, the routine CTSCAE is not called). Different values of 'CAF' may be used in a user version of CTSCAE to select different schemes for picking color indices.

IAID (INTEGER, input) is the value of the area identifier associated with the midpoint of the cell (IND1,IND2). The four possibilities are described above, in the description of the routine CTCICA.

CTSETC (PNAM,CVAL)

This routine is called to set the value of a parameter of type CHARACTER.

Usage

Use the statement 

      CALL CTSETC (PNAM,CVAL)
to give the parameter whose name is PNAM the character value CVAL. If that parameter is an array, the element specified by the current value of 'PAI' will be the one changed.

Arguments

PNAM (CHARACTER, input) is the name of a parameter to be given a character value. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of 'ILT', use 'ILT - INFORMATIONAL LABEL TEXT'.

CVAL (CHARACTER, input) is a character constant or variable, the value of which is to be given to the parameter specified by PNAM.

CTSETI (PNAM,IVAL)

This routine is called to give a new integer value to an internal parameter.

Usage

Use the statement

      CALL CTSETI (PNAM,IVAL)
to give the parameter whose name is PNAM the integer value IVAL. If that parameter is an array, the element specified by the current value of 'PAI' will be the one changed.

Arguments

PNAM (CHARACTER, input) is the name of a parameter to be given an integer value. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of 'ILA', use 'ILA - INFORMATIONAL LABEL ANGLE'.

IVAL (INTEGER, input) is an expression, the value of which is to be given to the parameter specified by PNAM. If the internal parameter is of type INTEGER, it is given the value IVAL; if the internal parameter is of type REAL, it is given the value REAL(IVAL).

CTSETR (PNAM,RVAL)

This routine is called to give a new real value to an internal parameter.

Usage

Use the statement 

      CALL CTSETR (PNAM,RVAL)
to give the parameter whose name is PNAM the real value RVAL. If that parameter is an array, the element specified by the current value of 'PAI' will be the one changed.

Arguments

PNAM (CHARACTER, input) is the name of a parameter to be given a real value. Only the first three characters of PNAM are examined. It is recommended that the rest of the character string be used to improve the readability of the code. For example, instead of 'CIS', use 'CIS - CONTOUR INTERVAL SPECIFIER'.

RVAL (REAL, input) is an expression, the value of which is to be given to the parameter specified by PNAM. If the internal parameter is of type INTEGER, it is given the value INT(RVAL); if the internal parameter is of type REAL, it is given the value RVAL.

CTTDBF (RPNT,IEDG,ITRI,RWRK,IWRK,IFLG,ATOL)

This routine assumes that the triangular mesh is being projected using the 3D package TDPACK. When called, it sets bits of the triangle-blocking flags for all triangles of the mesh that are not currently blocked by the user in such a way as to enable identifying those that are seen from the "wrong side", those that are seen nearly edge-on, and/or those that are partially or completely hidden by other triangles of the mesh.

Let the low-order seven bits of each triangle's blocking flag be numbered (from left to right) B6, B5, B4, B3, B2, B1, and B0; the bits are assigned as follows:

    B6 B5 B4 B3 B2 B1 B0
     \  \  \  \  \  \  \__ reserved for user blocking of triangle
      \  \  \  \  \  \____ right (or only) eye, triangle seen from the wrong side
       \  \  \  \  \______ right (or only) eye, triangle edge-on to the line of sight
        \  \  \  \________ right (or only) eye, triangle hidden by other triangles
         \  \  \__________ left eye, triangle seen from the wrong side
          \  \____________ left eye, triangle edge-on to the line of sight
           \______________ left eye, triangle hidden by other triangles
If the final argument (OTEP) in the last call to TDINIT (the offset to the eye position), was greater or equal to zero, then CTTDBF will set bits B3, B2, and B1 of each triangle blocking flag; if OTEP was less than zero, then CTTDBF will set bits B6, B5, and B4 of each triangle blocking flag. Bit B0 is not affected by the call, but the upper bits (B7 and above) are cleared for any triangle that is not blocked by the user.

The blocking flag allows one to maintain visibility information for all triangles of the mesh from two different viewpoints, which means that, by setting the triangle blocking mask parameters 'TBX' and 'TBA' appropriately, one can draw views from those two positions, on each view showing only the triangles visible from that viewpoint.

The argument IFLG allows the caller to say which conditions are to be tested for (as detailed below, in the section "Arguments"); when it is given the value 2 or 3, an algorithm is executed to determine which triangles are partially or completely hidden by other triangles. To run efficiently, this algorithm requires some integer workspace in IWRK; by default, it uses 2500 words, as specified by the value of the internal parameter 'IWB'; increasing the value of 'IWB' (and, of course, the declared length of IWRK) may make this algorithm run even faster. Some experimentation may be required to determine the optimum value to use.

See also the discussions of the internal parameters 'IWB', 'TBX', and 'TBA', the subroutine CTTDBM, and the examples "cttd01" and "cttd02".

Usage

The routine CTTDBF may be called at any time after the initialization call to CTMESH and the appropriate initialization calls to TDPACK.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

IFLG (INTEGER, input), is an expression, the value of which must be 0, 1, 2, or 3, with meanings as follows:

ATOL (REAL, input), is an expression, the value of which is a tolerance, in degrees, for the "edge-on" tests. If ATOL is less than or equal to zero, edge-on testing is turned off; otherwise, edge-on triangles are those whose center-point normals are within ATOL degrees of being perpendicular to the line of sight to that point.

CTTDBM (IHBX,IEBX,IWBX,IUBX,IHBA,IEBA,IWBA,IUBA)

This routine assumes that the triangular mesh is being projected using the 3D package TDPACK. When called, it sets the values of the internal parameters'TBX' and 'TBA', which are used as masks for the triangle blocking flags, in such a way as to achieve a particular desired effect.

Let the low-order seven bits of each triangle's blocking flag be numbered (from left to right) B6, B5, B4, B3, B2, B1, and B0; the bits are assigned as follows:

    B6 B5 B4 B3 B2 B1 B0
     \  \  \  \  \  \  \__ reserved for user blocking of triangle
      \  \  \  \  \  \____ right (or only) eye, triangle seen from the wrong side
       \  \  \  \  \______ right (or only) eye, triangle edge-on to the line of sight
        \  \  \  \________ right (or only) eye, triangle hidden by other triangles
         \  \  \__________ left eye, triangle seen from the wrong side
          \  \____________ left eye, triangle edge-on to the line of sight
           \______________ left eye, triangle hidden by other triangles
The user sets the B0 bits of the blocking flags directly; the routine CTTDBF is called to set the others. Subsequently, before drawing anything derived from the triangular mesh (contour lines, for example), a call to CTTDBF may be done to determine how the bits of the blocking flags are to be interpreted.

If the final argument (OTEP) in the last call to TDINIT (the offset to the eye position), was greater or equal to zero, CTTDBM will set bits B3, B2, B1, and B0 of 'TBX' and 'TBA', thus generating masks for the right (or only) eye; if OTEP was less than zero, CTTDBM will set bits B6, B5, B4, and B0 of 'TBX' and TBA', thus generating masks for the left eye.

Each bit of 'TBX' (an XOR mask) that is a "1" acts as a toggle for the corresponding bit of a triangle's blocking flag, reversing its meaning, while each bit of 'TBA' (an AND mask) that is a "1" acts as a selector for the corresponding bit of a triangle's blocking flag, selecting it as a meaningful bit for testing purposes.

For example, the call

C                x x x x a a a a
C                h e w u h e w u
    CALL CTTDBM (0,0,0,0,1,1,1,1)
says that no bits of the triangles' blocking flags are to be toggled and that all of the bits are to be capable of blocking the triangle. The effect will be to block all triangles that are hidden, edge-on, seen from the wrong side, or user-blocked.

The call

C                x x x x a a a a
C                h e w u h e w u
    CALL CTTDBM (0,0,0,0,1,1,0,1)
says that no bits of the triangles' blocking flags are to be toggled and that all of the bits except the "wrong-side" flag are to be capable of blocking the triangle. This allows one to (for example) draw the triangular-mesh edges, no matter which side of the mesh the triangles are seen from.

The call

C                x x x x a a a a
C                h e w u h e w u
    CALL CTTDBM (1,1,1,0,1,1,1,1)
says that the "hidden", "edge-on", and "wrong-side" bits of the triangles' blocking flags are to be toggled and that all of the bits in the flag are to be capable of blocking the triangle. This would allow one to use only those triangles that not blocked by the user, but are hidden, edge-on, or viewed from the wrong side. (It's not completely clear why one would want to do this, but it's possible to do it.)

See also the discussions of the internal parameters 'TBX' and 'TBA', the subroutine CTTDBF, and the examples "cttd01" and "cttd02".

Usage

The routine CTTDBF may be called at any time after the initialization call to CTMESH and the appropriate initialization calls to TDPACK.

Arguments

IHBX (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B6 or B3 of 'TBX'. This bit toggles the value of the "hidden" bit of the triangle's blocking flag.

IEBX (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B5 or B2 of 'TBX'. This bit toggles the value of the "edge-on" bit of the triangle's blocking flag.

IWBX (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B4 or B1 of 'TBX'. This bit toggles the value of the "wrong-side" bit of the triangle's blocking flag.

IUBX (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B0 of 'TBX'. This bit toggles the value of the "user" bit of the triangle's blocking flag.

IHBA (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B6 or B3 of 'TBA'. This bit masks the value of the "hidden" bit of the triangle's blocking flag.

IEBA (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B5 or B2 of 'TBA'. This bit masks the value of the "edge-on" bit of the triangle's blocking flag.

IWBA (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B4 or B1 of 'TBA'. This bit masks the value of the "wrong-side" bit of the triangle's blocking flag.

IUBA (INTEGER, input), is an expression, the value of which must be 0 or 1, which provides the value of bit B0 of 'TBA'. This bit masks the value of the "user" bit of the triangle's blocking flag.

CTTDCA (RPNT,IEDG,ITRI,RWRK,IWRK, ... )

(The remaining arguments are ICRA,ICA1,ICAM, ICAN,XCPF, YCPF, XCQF, and YCQF.)

This routine creates a GKS cell array, using color indices determined by examining where the user's contours lie relative to the cell array, just as the routine CTCICA does, but CTTDCA assumes that routines from the 3D package TDPACK are being used to project the 3-dimensional triangular mesh into a 2-dimensional image space. If the center of a given cell of the cell array is between the eye and more than one of the triangles of the triangular mesh, that triangle which is closest to the eye is the one used to determine the color of the cell, thus creating a perspective picture of the triangular mesh.

Unlike CTCICA, this routine examines all triangles of the triangular mesh except those that are explicitly blocked by the user; that is, it makes use of only the lowest-order bits of the blocking masks 'TBX' and 'TBA' and the blocking flag for each triangle. This allows it to paint a much better picture of the mesh than CTCICA can do.

See the examples "cttd01" and "cttd02".

Usage

The routine CTTDCA may be called at any time after the initialization call to CTMESH. It is given arrays defining a triangular mesh of data, a real workspace array, an integer workspace array, an array in which a GKS cell array is to be created, and dimensioning and positioning information for the cell array. It creates the cell array by associating with each cell of it an area identifier (let's call it IAID) and then using IAID to generate a color index to store in that cell; there are three possible values of IAID:

Note that, to be sure of distinguishing all three possibilities from each other, it is necessary to give elements -1 and -2 of the parameter array 'AIA' values which are different from the value of any other element of 'AIA' or 'AIB'.

Once an area identifier has been associated with a particular cell, that area identifier must be mapped into a color index to be stored as the value of the corresponding element of the cell array. The internal parameter 'CAF' determines how area identifiers are mapped into color indices, as follows:

The default value of 'CAF' is zero, so that CTSCAE is not called: if IAID is non-negative, it is used as the desired color index for a particular cell; otherwise, a zero is used.

Note that the routine CTCTCA works differently from the routine CPCICA, in CONPACK, which allowed one to modify some elements of an existing cell array, while leaving others unchanged. CPCICA could do that because the CONPACK routine CPMPXY implemented full inverse mappings, which the CONPACKT routine CTMXYZ does not do for the TDPACK mapping.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

ICRA (INTEGER array, dimensioned ICA1 by "n", where "n" is greater than or equal to the value of the argument ICAN, input/output) is the user's cell array.

ICA1 (INTEGER, input) is the first dimension of the FORTRAN array ICRA, which contains the user's cell array.

ICAM (INTEGER, input) is the first dimension of the user's cell array.

ICAN (INTEGER, input) is the second dimension of the user's cell array.

XCPF and YCPF (REAL, input) are the coordinates, in the fractional coordinate system, of a point P, which is the point at that corner of the rectangular area into which the cell array maps that is also a corner of the cell with indices (1,1).

XCQF and YCQF (REAL, input) are the coordinates, in the fractional coordinate system, of a point Q, which is the point at that corner of the rectangular area into which the cell array maps that is also a corner of the cell with indices (ICAM,ICAN).

CTTDDM (RPNT,IEDG,ITRI,RWRK,IWRK,IDIA)

This routine assumes that routines from the 3D package TDPACK are being used to project the 3-dimensional triangular mesh into a 2-dimensional image space and calls TDLINE repeatedly to draw that part of the mesh which is unblocked, where the blocking is as specified by the blocking masks 'TBX' and 'TBA' and the blocking flags for the triangles.

See the examples "cttd01" and "cttd02".

Usage

The routine CTTDDM may be called at any time after the initialization call to CTMESH. It is given arrays defining a triangular mesh of data, a real workspace array, an integer workspace array, and a flag that can be used to suppress certain portions of the mesh.

Arguments

The first five arguments are arrays used in a previous call to one of the routines CTMESH, CTMVRW, or CTMVIW.

RPNT (REAL array, dimensioned as specified in the last call to CTMESH, input) is the user's mesh-point array.

IEDG (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's edge array.

ITRI (INTEGER array, dimensioned as specified in the last call to CTMESH, input) is the user's triangle array.

RWRK (REAL array, dimensioned as specified in the last call to CTMESH or CTMVRW, input/output) is the current real workspace array.

IWRK (INTEGER array, dimensioned as specified in the last call to CTMESH or CTMVIW, input/output) is the current integer workspace array.

IDIA (INTEGER, input), if non-zero, is the index of an element in each edge node that acts as a flag to indicate whether that edge is to be drawn (zero) or not (non-zero). See the example mentioned above ("cttd01"), which uses this feature to suppress the drawing of those edges of the mesh that were diagonals of the quadrilaterals of the original mesh from which the triangular mesh was created. Note that, if this feature is used, code must be inserted to create the specified elements in the edge nodes.

CTTMRG (IDIM,JDIM,RLAT,RLON,RDAT, ... )

(The remaining arguments are ISCR, SVAL, RTMI, RPNT, MPNT, NPNT, LOPN, IEDG, MEDG, NEDG, LOEN, ITRI, MTRI, NTRI, and LOTN.)

The routine CTTMRG ("ConpackT, Triangular Mesh from Rectangular mesh on Globe") is intended to simplify the process of creating triangular meshes of a particular type: given arrays defining a rectangular grid of data deformed to wrap around the globe, it returns arrays defining a triangular mesh representing the data.

Usage

This routine is called using a FORTRAN statement like the following: 
        CALL CTTMRG (IDIM,JDIM,            !  dimensioning information
     +               RLAT,RLON,RDAT,ISCR,  !  data arrays, scratch array
     +               SVAL,RTMI,            !  special value, index mapper
     +               RPNT,MPNT,NPNT,LOPN,  !  point node array
     +               IEDG,MEDG,NEDG,LOEN,  !  edge node array
     +               ITRI,MTRI,NTRI,LOTN)  !  triangle node array

Arguments

IDIM (INTEGER, input) is the first dimension of the rectangular mesh.

JDIM (INTEGER, input) is the second dimension of the rectangular mesh.

RLAT and RLON (REAL arrays, dimensioned IDIM x JDIM, input) specify the latitudes and longitudes of the node points of the rectangular mesh. The mesh is the collection of quadrilaterals defined by corner points having indices (I,J), (I+1,J), (I,J+1), and (I+1,J+1), for all pairs (I,J) such that I is between 1 and IDIM-1, inclusive, and J is between 1 and JDIM-1, inclusive. The mesh may (and, indeed, probably will) touch itself along certain "seams", but it must not overlap itself.

RDAT (REAL array, dimensioned IDIM x JDIM, input) specifies the data values at the points of the rectangular mesh.

ISCR (INTEGER array, dimensioned IDIM x JDIM x 4, scratch) is a scratch array required by CTTMRG.

SVAL (REAL, input) is a value which, if used in the array RDAT, marks that datum as "special" or "missing". If there are no such values in the array RDAT, set SVAL to some value known not to occur in it.

RTMI ("Routine To Map Indices", user subroutine, input) is a user-defined subroutine describing how the edges of the rectangular mesh, as deformed onto the surface of the globe, fit together. RTMI allows CTTMRG to create a triangular mesh that contains only as many distinct points as there were different points in the rectangular mesh and that has no "seams" where the edges of the rectangular mesh came together.

RTMI must be declared EXTERNAL in the routine that calls CTTMRG; it will be called using FORTRAN statements like this:

        CALL RTMI (IDIM,JDIM,IINI,JINI,IINO,JINO)
The arguments IDIM and JDIM are as defined above. The arguments IINI and JINI are input expressions of type INTEGER defining the indices of a particular point of the rectangular mesh (where IINI is between 1 and IDIM and JINI is between 1 and JDIM, inclusive). The arguments IINO and JINO are output variables of type INTEGER that receive the values to be used for the specified point of the mesh in place of IINI and JINI. In general, IINO = IINI and JINO = JINI everywhere except at points that are coincident with other points of the mesh. For example, if the rectangular mesh wraps around the globe in such a way that the entire bottom and top edges of the mesh (for J = 1 and J = JDIM, respectively) each map into a single point (typically the south pole and the north pole, respectively) and the left and right edges of the mesh (for I = 1 and I = IDIM) are coincident on the globe, then one would define RTMI as follows:

      SUBROUTINE RTMI (IDIM,JDIM,IINI,JINI,IINO,JINO)
        IF (JINI.EQ.1) THEN          !  point in first row of mesh
          IINO=1
          JINO=1
        ELSE IF (JINI.EQ.JDIM) THEN  !  point in last row of mesh
          IINO=1
          JINO=JDIM
        ELSE IF (IINI.EQ.IDIM) THEN  !  point in last column of mesh
          IINO=1
          JINO=JINI
        ELSE                         !  all other points of the mesh
          IINO=IINI
          JINO=JINI
        END IF
        RETURN
      END
RPNT (REAL array, dimensioned greater than or equal to MPNT x LOPN, output) receives the point array.

MPNT (INTEGER, input) is the dimension of RPNT.

NPNT (INTEGER, output) is the number of elements returned in RPNT (that is, the number of points in the triangular mesh, times LOPN).

LOPN (INTEGER, input) is the length of a point node.

IEDG (INTEGER array, dimensioned greater than or equal to MEDG x LOEN, output) receives the edge array.

MEDG (INTEGER, input) is the dimension of IEDG.

NEDG (INTEGER, output) is the number of elements returned in IEDG (that is, the number of edges of the triangular mesh, times LOEN).

LOEN (INTEGER, input) is the length of an edge node.

ITRI (INTEGER array, dimensioned greater than or equal to NTRI x LOTN, output) receives the triangle array.

MTRI (INTEGER, input) is the dimension of ITRI.

NTRI (INTEGER, output) is the number of elements returned in ITRI (that is, the number of triangles of the triangular mesh, times LOTN).

LOTN (INTEGER, input) is the length of a triangle node.

CTTMTL (NTTO,TBUF,MBUF,NBUF, ... )

CTTMTX (NTTO,TBUF,MBUF,NBUF,EPST, ... )

(In each case, the remaining arguments are IPPP, MPPP, NPPP, IPPE, MPPE, NPPE, RPNT, MPNT, NPNT, LOPN, IEDG, MEDG, NEDG, LOEN, ITRI, MTRI, NTRI, and LOTN.)

The routines CTTMTL ("ConpackT, Triangular Mesh from Triangle List") and CTTMTX (" ..., eXtended") make it relatively easy to obtain, from an collection of triangles in 3-space, a triangular mesh in the form required by CONPACKT. Normally, of course, the collection isn't entirely arbitrary, since the triangles fit together to form a mesh.

One need not supply information specifying which pairs of triangles adjoin; CTTMTL and CTTMTX can figure that out for themselves. However, it must be the case that, when two triangles have an edge in common, they have the entire edge in common (i.e., there must be at most one triangle to the left of each edge and at most one triangle to the right of each edge).

CTTMTL requires that the coordinates of a vertex that two triangles have in common be computed exactly the same in both triangles. Sometimes, this is a difficult thing to ensure; in such cases, one may call CTTMTX instead and provide an additional argument, EPST, that defines what it means for two coordinate values to be "identical". Note that CTTMTX is a little slower and it requires a larger scratch array IPPP for use in sorting the points (because the tree-sort nodes must contain backward pointers as well as forward pointers).

Usage

CTTMTL and CTTMTX are called using FORTRAN code something like the following. (Note that the details may change from one program to another: for example, some of the DIMENSION statements could be in a calling routine and the various parameters could be passed as arguments.)

C
C Declare the maximum number of points, edges, and triangles expected.
C
        PARAMETER (MNOP=maximum_number_of_points,MPPP=MNOP)
        PARAMETER (MNOE=maximum_number_of_edges,MPPE=MNOE)
        PARAMETER (MNOT=maximum_number_of_triangles)
C
C Declare the lengths of a point node, an edge node, and a triangle
C node, respectively.
C
        PARAMETER (LOPN=4,LOEN=5,LOTN=4)
C
C Declare the lengths of the arrays in which the triangular mesh is to
C be formed.
C
        PARAMETER (MPNT=MNOP*LOPN)  !  space for points
        PARAMETER (MEDG=MNOE*LOEN)  !  space for edges
        PARAMETER (MTRI=MNOT*LOTN)  !  space for triangles
C
C Declare the arrays to hold the point nodes, edge nodes, and triangle
C nodes of the triangular mesh.
C
        DIMENSION RPNT(MPNT),IEDG(MEDG),ITRI(MTRI)
C
C Declare a triangle buffer in which to store triangles temporarily.
C This buffer makes it possible to randomize the order in which the
C triangles of the mesh are processed.  This is necessary to ensure
C friendly behavior by the tree sorts that are done to identify points
C and edges that more than one triangle have in common.  The size of
C the buffer (MBUF) and the number of triangles processed from it at
c a time when the buffer is full (KBUF) are dependent on the size of
C the user's triangular mesh.
C
        PARAMETER (MBUF=5021,KBUF=173)
C
        DIMENSION TBUF(12,MBUF)
C
C Declare a scratch array to be used to sort points.
C
        DIMENSION IPPP(2,MPPP)
C
C Declare a scratch array to be used to sort edges.
C
        DIMENSION IPPE(2,MPPE)
C
C Initialize various counters.
C
        NPPP=0  !  number of points in point sorter
        NPPE=0  !  number of edges in edge sorter
        NBUF=0  !  number of triangles in triangle randomizing buffer
        NTRI=0  !  number of triangles in final triangle list
C
C Loop on the triangles in the mesh.
C
        DO 101 I=1,number_of_triangles_in_arbitrary_mesh
C
C If the triangle buffer is full, process KBUF randomly-chosen
C triangles from it, leaving the remainder at the beginning of
C the buffer.
C
          IF (NBUF.GE.MBUF) THEN
            CALL CTTMTL (KBUF,                 !  number to process
     +                   TBUF,MBUF,NBUF,       !  triangle buffer
     +                   IPPP,MPPP,NPPP,       !  point sorting array
     +                   IPPE,MPPE,NPPE,       !  edge sorting array
     +                   RPNT,MPNT,NPNT,LOPN,  !  point node array
     +                   IEDG,MEDG,NEDG,LOEN,  !  edge node array
     +                   ITRI,MTRI,NTRI,LOTN)  !  triangle node array
          END IF
C
C Generate triangle I and put it in the triangle buffer.
C
          NBUF=NBUF+1
C
          TBUF( 1,NBUF)=X coordinate of point 1 of triangle I
          TBUF( 2,NBUF)=Y coordinate of point 1 of triangle I
          TBUF( 3,NBUF)=Z coordinate of point 1 of triangle I
          TBUF( 4,NBUF)=data value at point 1 of triangle I
C
          TBUF( 5,NBUF)=X coordinate of point 2 of triangle I
          TBUF( 6,NBUF)=Y coordinate of point 2 of triangle I
          TBUF( 7,NBUF)=Z coordinate of point 2 of triangle I
          TBUF( 8,NBUF)=data value at point 2 of triangle I
C
          TBUF( 9,NBUF)=X coordinate of point 3 of triangle I
          TBUF(10,NBUF)=Y coordinate of point 3 of triangle I
          TBUF(11,NBUF)=Z coordinate of point 3 of triangle I
          TBUF(12,NBUF)=data value at point 3 of triangle I
C
  101   CONTINUE
C
C Process any triangles that remain in the triangle buffer.
C
        IF (NBUF.NE.0) THEN
          CALL CTTMTL (NBUF,                 !  number to process
     +                 TBUF,MBUF,NBUF,       !  triangle buffer
     +                 IPPP,MPPP,NPPP,       !  point sorting array
     +                 IPPE,MPPE,NPPE,       !  edge sorting array
     +                 RPNT,MPNT,NPNT,LOPN,  !  point node array
     +                 IEDG,MEDG,NEDG,LOEN,  !  edge node array
     +                 ITRI,MTRI,NTRI,LOTN)  !  triangle node array
        END IF
Note that, if the triangle buffer is large enough to hold all the triangles of the mesh at once, the first call to CTTMTL and its enclosing block-IF can be omitted, since all the triangles will be processed out of the buffer by the second call to CTTMTL.

Arguments

NTTO (INTEGER, input) is the number of triangles that CTTMTL is to process from TBUF.

TBUF (REAL array, dimensioned 12 x MBUF, input) is an array of triangles that are to be used to create a triangular mesh. For each J from 1 to NBUF, the elements of TBUF(I,J), for I from 1 to 12, specify a triangle, as follows: TBUF(1,J) through TBUF(3,J) are the X, Y, and Z coordinates of point 1 and TBUF(4,J) is a data value associated with point 1; similarly, TBUF(5,J) through TBUF(8,J) define point 2 and TBUF(9,J) through TBUF(12,J) define point 3.

MBUF (INTEGER, input) is the second dimension of the array TBUF.

NBUF (INTEGER, input/output), is the number of triangles defined by the current contents of TBUF.

EPST (REAL, input, CTTMTX only), is an epsilon to be used in testing for equality of point coordinate values. Two coordinate values will be considered identical if the absolute value of the difference between them is less than or equal to EPST.

IPPP (INTEGER array, dimensioned 2 x MPPP (if CTTMTL is called) or 3 x MPPP (if CTTMTX is called), scratch) is a scratch array required by CTTMTL to sort points.

MPPP (INTEGER, input) is the second dimension of IPPP, which must be greater than or equal to the total number of distinct points in the triangular mesh.

NPPP (INTEGER, input/output) is the number of elements of IPPP used so far.

IPPE (INTEGER array, dimensioned 2 x MPPE, scratch) is a scratch array required by CTTMTL to sort edges.

MPPE (INTEGER, input) is the second dimension of IPPE, which must be greater than or equal to the total number of distinct edges in the triangular mesh.

NPPE (INTEGER, input/output) is the number of elements of IPPE used so far.

RPNT (REAL array, dimensioned greater than or equal to MPNT x LOPN, output) receives the point array.

MPNT (INTEGER, input) is the dimension of RPNT.

NPNT (INTEGER, output) is the number of elements returned in RPNT (that is, the number of points in the triangular mesh, times LOPN).

LOPN (INTEGER, input) is the length of a point node.

IEDG (INTEGER array, dimensioned greater than or equal to MEDG x LOEN, output) receives the edge array.

MEDG (INTEGER, input) is the dimension of IEDG.

NEDG (INTEGER, output) is the number of elements returned in IEDG (that is, the number of edges of the triangular mesh, times LOEN).

LOEN (INTEGER, input) is the length of an edge node.

ITRI (INTEGER array, dimensioned greater than or equal to NTRI x LOTN, output) receives the triangle array.

MTRI (INTEGER, input) is the dimension of ITRI.

NTRI (INTEGER, output) is the number of elements returned in ITRI (that is, the number of triangles of the triangular mesh, times LOTN).

LOTN (INTEGER, input) is the length of a triangle node.

PARAMETERS

The behavior of CONPACKT is controlled by a large number of parameters in internal common blocks to which all of its routines have access. These parameters are described in detail in this section.

Parameter Names

Each of the parameters of CONPACKT is identified by a three-character mnemonic name. For example, 'NCL' is the name of the parameter specifying the number of contour levels.

Parameter Types

Each parameter is intrinsically of type CHARACTER, INTEGER, or REAL, but the type of the parameter is not implied by the form of its name; one must read the description of the parameter to determine its type.

Parameter Defaults

Each parameter has a default value which is intended to yield a reasonable, useful, default behavior. To modify the behavior of CONPACKT routines, one changes the values of the appropriate parameters at the appropriate time.

Parameter Access

The value of a parameter may be set using one of the three routines CTSETC, CTSETI, and CTSETR. Each of these routines has as its first argument a character string beginning with the three-character name of the parameter and as its second argument an expression of the type implied by the last character of the routine name ("C" for "CHARACTER", "I" for "INTEGER", or "R" for "REAL"). Similarly, the current value of a parameter may be retrieved using one of the three routines CTGETC, CTGETI, and CTGETR, each of which has as its first argument the name of the parameter and as its second argument a variable of the type implied by the last character of the routine name.

In general, once a parameter is given a particular value, it retains that value until it is given a new value; with only a couple of exceptions, there is no automatic resetting of parameters at any time.

Parameter-Name Arguments

Only the first three characters of the parameter-name argument in calls to the parameter access routines are examined. It is strongly recommended that additional characters be added in order to make the code more readable. For example, a call to set the number of contour levels might read

      CALL CTSETI ('NCL - NUMBER OF CONTOUR LEVELS',13)
The additional characters make it much easier to remember what the call is intended to do.

Automatic Type Conversion

Normally, one would use CTSETI to set parameters of type INTEGER and CTSETR to set parameters of type REAL. However, since automatic conversion is done as necessary, this need not be the case. One could, for example, use either of the two statements

      CALL CTSETI ('ILA - INFORMATIONAL LABEL ANGLE',30)
      CALL CTSETR ('ILA - INFORMATIONAL LABEL ANGLE',30.)
to set the informational-label angle (which is intrinsically REAL) to 30 degrees. The first has the virtue of being one character shorter. Similarly, one could use either of the two statements

      CALL CTSETI ('NCL - NUMBER OF CONTOUR LEVELS',18)
      CALL CTSETR ('NCL - NUMBER OF CONTOUR LEVELS',18.)
to set the number of contour levels (which is intrinsically INTEGER) to 18. (In this case, of course, the first of the two statements seems to make better sense.) If a REAL parameter is to be given a non-integral value (like 3.14159), then CTSETR must be used, of course.

Certain parameters, of type CHARACTER, representing dash patterns, may be set using either a call to CTSETC or CTSETI; in the latter case, the low-order sixteen bits of the integer are interpreted and used to construct a character dash pattern (by mapping 0's into apostrophes and 1's into dollar signs).

Automatic Restriction of Parameter Values

Some parameters may take on any possible value of the proper type: for example, 'CFA', which specifies the angle at which the constant-field label is to be written, may be given any real value. Other parameters may only be given values in a restricted range: for example, 'CFB', which specifies whether the constant-field label is to be boxed, and, if so, in what manner, is restricted to the integer values 0, 1, 2, and 3. The parameter-setting routines attempt to enforce such restrictions. Attempting to set 'CFB' to a non-integral value will result in truncation of the fractional part; attempting to give it a value less than 0 will result in giving it the value 0 and attempting to give it a value greater than 3 will result in giving it the value 3. Other parameters are treated similarly.

Parameter Arrays

Some of the parameters are actually arrays. For example, the parameter 'CLV', which specifies contour levels, is actually a 256-element array. In order to access the Ith element of such a parameter array, one must somehow specify the value of the index I. This is done by making one parameter, named 'PAI', serve as a "parameter array index". Thus, to set the tenth contour level to 196.3, one would use the following code:

      CALL CTSETI ('PAI - PARAMETER ARRAY INDEX',10)
      CALL CTSETR ('CLV - CONTOUR LEVEL VALUE',196.3)
In a few cases, negative values of PAI must be used. A negative value of PAI is not used directly as an index value; it merely serves as a signal to the parameter-access routines that a special element of the parameter array is being accessed. These cases will be pointed out as they are encountered.

Parameter Descriptions

Parameter descriptions follow, in alphabetical order. Each description begins with a line giving the three-character mnemonic name of the parameter, the phrase for which the mnemonic stands, the intrinsic type of the parameter, and an indication of whether or not it is an array.

'AIA' - Area Identifier Above - Integer Array

Each element of the parameter array 'AIA' is an area identifier for the area above the contour level specified by the corresponding element of the parameter array 'CLV'. The corresponding element of the parameter array 'AIB' is an area identifier for the area below that level. If, for a particular contour level, both 'AIA' and 'AIB' are zero, that level is ignored by the routine CTCLAM; otherwise, contour lines at that level are added to the area map and the given values of 'AIA' and 'AIB' are used as right and left area identifiers.

There are two special elements in the parameter array 'AIA', corresponding to 'PAI' values of -1, and -2; the first specifies an area identifier for the area outside the edge of the grid and the second an area identifier for any area in which the mapping routine CTMXYZ returns the "out of range" value 'ORV'.

If contour levels are chosen automatically, rather than being supplied by the user, the value supplied for the Ith element of 'AIA' is I+1 and the value supplied for the Ith element of 'AIB' is I.

The default value of element "-1" of 'AIA' is 0 and the default value of element "-2" is -1.

'AIB' - Area Identifier Below - Integer Array

See the description of 'AIA', above.

'CAF' - Cell Array Flag - Integer

The parameter 'CAF' determines the way in which the routine CTCICA (which is called to store color indices in a user cell array), computes, from an area identifier associated with a particular cell, a color index for that cell. Let IAID be the area identifier that is associated with a given cell. Then:

The default value of 'CAF' is 0.

'CFA' - Constant-Field Label Angle - Real

The parameter 'CFA' specifies the angle (in degrees counterclockwise from a vector pointing to the right) at which a constant-field label is to be written.

The default value of 'CFA' is 0.

'CFB' - Constant-Field Label Box Flag - Integer

If 'CFB' is zero, the constant-field label will not be boxed at all. The value 1 implies that the perimeter of the box is to be drawn (in the same color as the label) after the label is drawn. The value 2 implies that the box is to be filled (in the color specified by 'LBC') before the label is drawn. The value 3 implies both of the above.

The default value of 'CFB' is 0.

'CFC' - Constant-Field Label Color Index - Integer

If 'CFC' is less than zero, the constant-field label and the box, if any, around it, will be drawn in the color specified by the current text color index; if 'CFC' is greater than or equal to zero, then it specifies the desired color index for the label and the box. If a box is drawn around the label, it is made the same color as the label itself.

The default value of 'CFC' is -1.

'CFF' - Constant-Field-Found Flag - Integer

The parameter 'CFF' may not be set by the user; its retrieved value will be non-zero if and only if CTMESH detected a constant field.

The default value of 'CFF' (prior to any call to CTMESH) is zero.

'CFL' - Constant-Field Label Line Width - Real

If 'CFL' is less than or equal to zero, line width will not be set before drawing a box around the constant-field label. If 'CFL' is greater than zero, it specifies the desired width, as a multiple of the "normal" line width.

The default value of 'CFL' is 0.

'CFP' - Constant-Field Label Positioning Flag - Integer

The parameter 'CFP' says how the constant-field label is to be positioned. There are nine possible values, each of which specifies a point of the label box which is to lie on the point defined by 'CFX' and 'CFY': the value -4 implies the lower left-hand corner of the label box, -3 implies the center of the bottom of the box, -2 the lower right-hand corner of the box, -1 the center of the left edge of the box, 0 the center of the box, +1 the center of the right edge of the box, +2 the upper left-hand corner of the box, +3 the center of the top edge of the box, and +4 the upper right-hand corner of the box. Left, right, bottom, and top are defined from the viewpoint of someone viewing the label right-side up.

The default value of 'CFP' is 0, so the constant-field label will be centered on the point whose coordinates are 'CFX' and 'CFY'.

'CFS' - Constant-Field Label Size - Real

The parameter 'CFS' specifies the nominal size (width) of a character in the constant-field label, as a fraction of the width of the viewport. This nominal size is multiplied by 'CWM'.

The default value of 'CFS' is .012.

'CFT' - Constant-Field Label Text String - Character

The parameter 'CFT' specifies the text of the constant-field label, which is written when a constant data field is detected; it is a character string of at most 64 characters. The embedded string '$DVA$' will be replaced by the numeric value of the field.

If 'CFT' is given the value ' ' (a single blank), the constant-field label will not be written.

The default value of 'CFT' is 'CONSTANT FIELD - VALUE IS $DVA$'.

'CFW' - Constant-Field Label White Space Width - Real

The parameter 'CFW' specifies the nominal width of white space to be left around the constant-field label, as a fraction of the width of the viewport. This nominal width is multiplied by 'CWM'.

The default value of 'CFW' is .005.

'CFX' - Constant-Field Label X Coordinate - Real

The parameter 'CFX' specifies the X coordinate of the basepoint of the constant-field label. The given value is mapped linearly onto the viewport; 0 refers to the left edge of the viewport and 1 to the right edge of the viewport. Values less than 0 or greater than 1 may be used.

The default value of 'CFX' is .5, so the constant-field label is centered horizontally in the viewport.

'CFY' - Constant-Field Label Y Coordinate - Real

The parameter 'CFY' specifies the Y coordinate of the basepoint of the constant-field label. The given value is mapped linearly onto the viewport; 0 refers to the bottom edge of the viewport and 1 to the top edge of the viewport. Values less than 0 or greater than 1 may be used.

The default value of 'CFY' is .5, so the constant-field label is centered vertically in the viewport.

'CIS' - Contour Interval Specifier - Real

See the description of 'CLS', below. When 'CLS' is greater than zero, 'CIS' is used. A value of 'CIS' less than or equal to zero indicates that CONPACKT is to choose the contour interval (see the descriptions of the parameter arrays 'CIT' and 'LIT'). A value of 'CIS' which is greater than zero is the actual contour interval to be used; in this case, 'LIS' may be given a non-zero value "n" to specify that every "nth" contour level should be labelled. See also the descriptions of 'CMN' and 'CMX', below.

The default value of 'CIS' is 0.

'CIT' - Contour Interval Table - Real Array

Each non-zero element of the ten-element parameter array 'CIT' is a real number greater than or equal to 1 and less than 10; when CONPACKT picks the contour interval, it chooses a number of the form "c x 10k", where "c" is one of the elements of 'CIT' and "k" is an integer. The non-zero elements of 'CIT' must be sorted in ascending order and appear at the beginning of the array.

The corresponding elements of the parameter array 'LIT' specify which contours are to be labelled. A contour at "nc x 10k" is labelled if "n" is a multiple of "l", where "l" is the element of 'LIT' corresponding to the element "c" of 'CIT'. For example, if the first element of 'CIT' is "1." and the first element of 'LIT' is "5", then CONPACKT is allowed to use contour levels 1., 2., 3., 4., etc., with labels at 5., 10., 15., etc. (5 x 1., 5 x 2., 5 x 3., etc.).

The default contents of 'CIT' and 'LIT' are as follows:

'PAI' 'CIT' 'LIT' LEVELS ALLOWED LABEL INTERVAL
1 1.0 5 1.0 x 10K EVERY 5TH ONE
2 2.0 5 2.0 x 10K EVERY 5TH ONE
3 2.5 4 2.5 x 10K EVERY 4TH ONE
4 4.0 5 4.0 x 10K EVERY 5TH ONE
5 5.0 5 5.0 x 10K EVERY 5TH ONE
6 0.0 0 none none
7 0.0 0 none none
8 0.0 0 none none
9 0.0 0 none none
10 0.0 0 none none

'CIU' - Contour Interval Used - Real

The parameter 'CIU' is normally intended for retrieval only. When the selection of the contour interval is left up to CONPACKT, 'CIU' is given the value chosen. When contour levels are completely set by the user, the value of 'CIU' may need to be set as well, for two reasons: 1) to make the desired value appear in an informational label (in place of the embedded string '$CIU$') and 2) so that it may be used by the penalty scheme for positioning labels. The setting of 'CIU' must be done after setting the contour levels (because, as a side effect of the setting of element 1 of 'CLV', 'CIU' is zeroed). If the user supplies contour levels, but supplies no value of 'CIU', and the penalty scheme is used to position labels, the required contour interval is estimated; in certain situations, this can lead to problems (if, for example, the same contour level appears twice in 'CLV', once to force lines at that level to be drawn and once to force that level to be used as the boundary for a shaded area).

The default value of 'CIU' is 0.

'CLC' - Contour Line Color Index - Integer Array

Each element of the parameter array 'CLC', if greater than or equal to zero, is a color index for contour lines at the level specified by the associated element of 'CLV'. A value less than zero implies that the lines will be drawn in the color specified by the current polyline color index.

There are two special elements in the parameter array 'CLC', corresponding to 'PAI' values of -1 and -2; the first specifies a color index for the edge of the grid and the second a color index for the edge of any area in which the mapping routine CTMXYZ returns the "out of range" value.

The default value of each element of 'CLC' is -1.

'CLD' - Contour Line Dash Pattern - Character Array

Each element of the parameter array 'CLD' is a dash pattern (as expected by the package DASHCHAR, when 'DPU' is greater than zero, or by the package DASHPACK, when 'DPU' is less than zero) to be used (when 'DPU' is non-zero) to draw contour lines at the level specified by the associated element of the contour level array 'CLV'. Elements of 'CLD' may be set using a call to CTSETI, with a sixteen-bit integer as the second argument, or using a call to CTSETC, with a character string of 128 or fewer characters as the second argument. In either case, the result will be a character string internally; a sixteen-bit integer will be converted to a sixteen-character string by mapping 0-bits into apostrophes and 1-bits into dollar signs. (When 'DPU' is negative, setting 'CLD' by means of a call to CTSETI will probably be inappropriate, since, by default, DASHPACK expects underscores, rather than apostrophes, to represent gaps in the dash pattern.)

There are two special elements in the parameter array 'CLD', corresponding to 'PAI' values of -1 and -2; the first specifies a dash pattern for the edge of the grid and the second a dash pattern for the edge of any area in which the mapping routine CTMXYZ returns the "out of range" value.

When CONPACKT picks the contour levels, the default value supplied for each associated dash pattern is the character constant '$$$$$$$$$$$$$$$$'. This is also the default value for each of the two special elements.

'CLL' - Contour Line Line Width - Real Array

Each element of the parameter array 'CLL' specifies the line width used to draw contour lines at the level specified by the associated element of the contour level array 'CLV'. Each is expressed as a multiple of the "normal" line width; values less than or equal to zero imply that line width should not be set.

There are two special elements in the parameter array 'CLL', corresponding to 'PAI' values of -1 and -2; the first specifies a line width for the edge of the grid and the second a line width for the edge of any area in which the mapping routine CTMXYZ returns the "out of range" value.

When CONPACKT picks the contour levels, the default value supplied for each associated line width is 0. This is also the default value for each of the two special elements.

'CLS' - Contour Level Selection Flag - Integer

This parameter must be set prior to the call to CTMESH which initiates the process of drawing a particular contour plot; it specifies how contour levels are to be selected, as follows:

The default value of 'CLS' is 16.

'CLU' - Contour Level Use Flags - Integer Array

Each element of the parameter array 'CLU' indicates how the associated contour level, in the parameter array 'CLV', is to be used. The value 0 means that no contour line is to be drawn at the associated level, the value 1 that the line is to be drawn without labels, the value 2 that the labels are to be drawn, but not the line, and the value 3 that both the line and the labels are to be drawn.

There are two special elements in the parameter array 'CLU', corresponding to 'PAI' values of -1 and -2; the first specifies a flag for the edge of the grid and the second a flag for the edge of any area in which the mapping routine CTMXYZ returns the "out of range" value. In each case, if the flag is zero, the associated edge is not drawn; otherwise, the associated edge is drawn.

When CONPACKT chooses the contour levels, the associated elements of 'CLU' are given one of the two values 1 or 3, depending on whether the line is to be labelled or not. The default values of the two special elements are zeroes.

'CLV' - Contour Level Values - Real Array

Each of the first 'NCL' elements of the parameter array 'CLV' is a contour level for which something is to be done (the drawing of contour lines, the drawing of contour labels, and/or the addition of contour lines to an area map).

Only elements 1 through 'NCL' may be accessed via the parameter-setting routines. Thus, code to set the contour levels and associated quantities must begin with a call to set 'NCL'.

A side effect of setting the element numbered 'PAI' of 'CLV' is that the associated element number 'PAI' in each of the parameter arrays 'AIA', 'AIB', 'CLC', 'CLD', 'CLL', 'CLU', 'LLC', and 'LLT' is also given a default value. These values are as follows:

PARAMETER DEFAULT VALUE
'AIA' 'PAI'+1
'AIB' 'PAI'
'CLC' -1
'CLD' '$$$$$$$$$$$$$$$$'
'CLL' 0
'CLU' 1
'LLC' -1
'LLT' ' ' (a single blank)

Thus, in code to set contour levels and associated quantities, each contour level must be set before the quantities associated with it.

A side effect of setting element number 1 of 'CLV' is that the parameter 'CIU', which indicates what contour interval was used, is zeroed. It is assumed that this will only happen when the user is providing all the contour levels, in which case the concept of the "contour interval" may or not be well defined. See the description of 'CIU' for more information.

'CMN' - Contour Minimum - Real

When 'CLS' is greater than zero and 'CIS' is also greater than zero, if 'CMN' is less than or equal to 'CMX', then the contour levels used will be of the form 'CMN', 'CMN'+'CIS', 'CMN'+2*'CIS', ... , 'CMN'+n*'CIS', where "n" is the largest integer such that 'CMN'+n*'CIS' is less than or equal to 'CMX'. The labelled levels will be those for which "n" is a multiple of 'LIS'.

The default values of 'CMN' and 'CMX' are 1 and 0, respectively.

'CMX' - Contour Maximum - Real

See the description of 'CMN', above.

'CTM' - Character Temporary - Character

The parameter name 'CTM' refers to a temporary character buffer in CONPACKT; the name may be used in the routine CTCHCL to get the dash pattern for the current line and in the routines CTCHHL, CTCHIL, and CTCHLL to get the text of the label being written or to change it.

The parameter 'CTM' has no meaningful default value.

'CWM' - Character Width Multiplier - Real

All character size parameters are multiplied by 'CWM'; this makes it easy to scale all the sizes up and down together. Parameters affected by this are 'CFS', 'CFW', 'DPS', 'DPV', 'HLS', 'HLW', 'ILS', 'ILW', 'LLS', and 'LLW'.

The default value of 'CWM' is 1.

'DMN' - Data Minimum Value - Real

The minimum value of the field data in the mesh, as found by CTMESH. For output only.

'DMX' - Data Maximum Value - Real

The maximum value of the field data in the mesh, as found by CTMESH. For output only.

'DPS' - Dash Pattern Size - Real

The parameter 'DPS' specifies the nominal size (width) of a character in a label in a dash pattern, as a fraction of the width of the viewport. This nominal size is multiplied by 'CWM'.

The default value of 'DPS' is .010.

'DPU' - Dash Pattern Use Flag - Integer

If 'DPU' is zero, no dash patterns are to be used and contour lines are to be drawn using calls to CURVE.

If 'DPU' is non-zero, dash patterns are to be used: if 'DPU' is greater than zero, contour lines are to be drawn using calls to the DASHCHAR routine CURVED, but if 'DPU' is less than zero, contour lines are to be drawn using calls to the DASHPACK routine DPCURV.

When the label positioning flag ABS('LLP') = 1, contour lines are caused to be labelled by using a dash pattern formed by concatenating "n" repetitions of the appropriate element of 'CLD' (the nominal dash pattern for the line) and the appropriate element of 'LLT' (the numeric label for the line); in this case, the absolute value of 'DPU' specifies the value of "n". When 'DPU' is less than zero and 'NET' = 1, numeric labels generated by CONPACKT will contain function codes that are meaningful to PLCHHQ; if it is desired to make these numeric labels bend with the curve they label, "break" characters must be inserted.

The default value of 'DPU' is 3.

'DPV' - Dash Pattern Vector Length - Real

The parameter 'DPV' specifies the nominal length of the solid vectors and the gap vectors resulting from the use of a dash pattern, as a fraction of the width of the viewport. This nominal length is multiplied by 'CWM'.

The default value of 'DPV' is .005.

'DVA' - Data Value - Real

The parameter 'DVA' is mostly for output. Its value may be retrieved in a user version of CTCHHL to retrieve the value of the high or low which is being labelled or in a user version of CTCHLL to retrieve the value associated with the contour line being labelled. If a character string representing the value is desired, CTGETC may be used to obtain it (as modified by the current scale factor); thus, to obtain the character representation of an arbitrary value in a form consistent with the other values on a contour plot, set 'DVA' with a call to CTSETR and retrieve the value of 'DVA' with a call to CTGETC; if an unscaled value is desired, use the parameter name 'DVU' in the call to CTGETC.

'DVU' - Data Value, Unscaled - Real

The parameter 'DVU' is just like 'DVA' (which see, above), but the value is unscaled.

'GIC' - Group Identifier for Contour Lines - Integer

The parameter 'GIC' specifies the group identifier for contour lines added to an area map by the routine CTCLAM. (CTCLAM also adds some other types of lines to the group with group identifier 'GIC': the edge of the current viewport; the edge of the grid; the edges of special-value areas, if any; and the edges of out-of-range areas, if any.)

The default value of 'GIC' is 3.

'GIL' - Group Identifier for Label Boxes - Integer

The parameter 'GIL' specifies the group identifier for label boxes added to an area map by the routine CTLBAM.

The default value of 'GIL' is 3.

'GIS' - Group Identifier for Strips - Integer

The parameter 'GIS' specifies the group identifier for a group of edges added to an area map by the routine CTCLAM to create a set of vertical strips. This is done only if the parameter 'NVS' (which is described later in this section) is non-zero.

The default value of 'GIS' is 4.

'HCF' - Hachuring Flag - Integer

The parameter 'HCF' is the hachuring flag, with one of the following values:

Negative values of 'HCF' may also be used. Each has the same effect as the corresponding positive value, except that the word "downhill" in the definition above is replaced by the word "uphill".

The default value of 'HCF' is 0.

The user will be expected to increase the value of the internal parameter 'RWC' ("Real Workspace for Contours") from its default 100 to a value large enough to accommodate any contour that can arise from his/her data field; this may require increasing the size of the real workspace array. (This is so that the hachuring routine is assured of seeing all of each contour line. Otherwise, it can't tell which way the "inside" and the "outside" of the closed contours are.)

Closed contours that are broken into pieces that appear to be open contours (either because portions of the closed contour disappear as a result of the mapping implied by non-zero values of the parameter 'MAP' and the out-of-range value 'ORV', or because they run through special-value areas, as defined by the internal parameter 'SPV', or because the user has forgotten to increase the value of 'RWC', as described in the previous paragraph), will be hachured. The rationale for this is that no real confusion can arise from hachuring a contour line which shouldn't be, only from not hachuring one that should be.

'HCL' - Hachure Length - Real

The parameter 'HCL' specifies the hachure length, stated as a fraction of the width of the viewport. A positive value implies the use of hachures on the downslope side of the contour. A negative value implies the use of hachures on the upslope side of the contour.

The default value of 'HCL' is .004.

'HCS' - Hachure Spacing - Real

The parameter 'HCS' specifies the hachure spacing, stated as a fraction of the width of the viewport. This is the distance between one hachure and the next along a contour line. Values outside the range [.0001,10.] are forced to the nearer end of that range.

The default value of 'HCS' is .01.

'HIC' - High Label Color Index - Integer

The parameter 'HIC' is used in determining the color index for high labels. See the description of 'HLC', below.

'HIT' - High Label Text String - Character

The parameter 'HIT' specifies the text string to be used in labelling a high. See the description of 'HLT', below.

'HLA' - High/Low Label Angle - Real

The parameter 'HLA' specifies the angle (in degrees counterclockwise from a vector pointing to the right) at which high and low labels are to be written.

The default value of 'HLA' is 0.

'HLB' - High/Low Label Box Flag - Integer

If 'HLB' is zero, high and low labels will not be boxed at all. The value 1 implies that the perimeter of the box is to be drawn (in the same color as the label) after the label is drawn. The value 2 implies that the box is to be filled (in the color specified by 'LBC') before the label is drawn. The value 3 implies both of the above.

The default value of 'HLB' is 0.

'HLC' - High/Low Label Color Index - Integer

The parameter 'HLC' is used in determining the color index for high and low labels.

The color index for high labels is determined in this manner: If 'HIC' is greater than or equal to zero, 'HIC' is used as the color index. If 'HIC' is less than zero, but 'HLC' is greater than or equal to zero, 'HLC' is used as the color index. If both 'HIC' and 'HLC' are less than zero, the current text color index is used. If a box is drawn around the label, it is made the same color as the label itself.

The color index for low labels is determined similarly: If 'LOC' is greater than or equal to zero, 'LOC' is used as the color index. If 'LOC' is less than zero, but 'HLC' is greater than or equal to zero, 'HLC' is used as the color index. If both 'LOC' and 'HLC' are less than zero, the current text color index is used. If a box is drawn around the label, it is made the same color as the label itself.

To set the color index of all high and low labels, simply supply the desired value for 'HLC'. To have highs and low labels which are colored differently, set 'HIC' and 'LOC'.

The default values of 'HLC', 'HIC', and 'LOC' are all -1's.

'HLE' - High/Low Equal-Value Search - Integer

If 'HLE' has the value zero, only the "normal" search for highs and lows, as described above in the section "Searching for Highs and Lows", is performed.

If 'HLE' has the value one, then the additional search is performed. See the subsection "Extended High/Low Search Algorithm", in the section "Searching for Highs and Lows", above.

If 'HLE' has the value two or greater, then the additional search is performed, but the candidate regions considered are limited to those containing no more than 'HLE' grid points.

Whenever 'HLE' is given a non-zero value, care should be taken to provide enough space in the integer work array to hold an additional N elements, where N is the number of points in the triangular mesh. This space is needed to implement the additional search algorithm.

The default value of 'HLE' is zero.

'HLL' - High/Low Line Width - Real

If 'HLL' has a value less than or equal to zero, line width will not be set before drawing boxes around high and low labels. If 'HLL' has a value greater than zero, it specifies the desired width, as a multiple of the "normal" line width.

The default value of 'HLL' is 0.

'HLO' - High/Low Label Overlap Flag - Integer

The value of 'HLO' says what is to be done about the problem of high and low labels overlapping other objects. The value 0 implies that the problem will be ignored; high and low labels will not be checked for overlap with anything else. Adding 1 to the value of 'HLO' implies the omission of high and low labels which overlap the informational label. Adding 2 implies the omission of high and low labels which overlap other high and low labels found before it. Adding 4 implies the omission of high and low labels which overlap the edges of the viewport, while adding 8 implies that high and low labels which overlap the edges of the viewport should be moved inward by just enough to take care of the problem. If you add both 4 and 8, the effect will be as if you had added 4 alone.

The default value of 'HLO' is 3 (1 + 2).

'HLR' - High/Low Search Radius - Real

The parameter 'HLR' specifies the radius of a sphere around a possible high (low) within which field values must be less than (greater than) the value at the possible high (low) in order for it to be marked. A positive value specifies a radius equal to 'HLR'*MAX('XMX'-'XMN', 'YMX'-'YMN','ZMX'-'ZMN'), while a negative value specifies the value ABS('HLR').

The default value of 'HLR' is .075.

'HLS' - High/Low Label Size - Real

The parameter 'HLS' specifies the nominal size (width) of a character in a high or low label, as a fraction of the width of the viewport. This nominal size is multiplied by 'CWM'.

The default value of 'HLS' is .012.

'HLT' - High/Low Label Text Strings - Character

The character strings used to label highs and lows may be specified individually, by setting 'HIT' and 'LOT', or together, by setting 'HLT'. If 'HLT' is set, and there are no apostrophes in the given character string, both 'HIT' and 'LOT' will be set equal to it and it will therefore be used as the label for both highs and lows. If there are apostrophes in the string, what precedes the first one will be used as the value of 'HIT' (the label for a high) and what follows it will be used as the value of 'LOT' (the label for a low).

Remember that, in FORTRAN, an apostrophe in a string which is delimited by apostrophes is represented by two apostrophes.

The substring $DVA$ may be used to represent the numeric value of the high or the low, divided by the current scale factor; the substring $DVAU$ may be used to represent the unscaled value.

Some examples:

FORTRAN STRING HIGH LABEL LOW LABEL
'H''L' H L
'HI''LO' HI LO
'$DVA$' 1.362 .764
'H($DVA$)''L($DVA$)' H(1.362) L(.764)
'H:B:$DVA$:E:''L:B:$DVA$:E:' H1.362 L.764

Note that, in the final example, the subscripting capability of the utility PLOTCHAR is used. The terminating function code "E" ensures that the "H" or the "L" will be centered on the high or low; to center the whole thing, either remove the 'E's or change them to 'N's.

Neither of the character strings 'HIT' and 'LOT' may contain more than 32 characters.

If 'HIT' is blank, highs will not be labelled. If 'LOT' is blank, lows will not be labelled.

The default value for 'HIT' is 'H:B:$DVA$:E:' and the default value of 'LOT' is 'L:B:$DVA$:E:', as shown in the final example above.

'HLW' - High/Low Label White Space Width - Real

The parameter 'HLW' specifies the nominal width of white space to be left around a high or low label, as a fraction of the width of the viewport. This nominal width is multiplied by 'CWM'.

The default value of 'HLW' is .005.

'ILA' - Informational Label Angle - Real

The parameter 'ILA' specifies the angle (in degrees counterclockwise from a vector pointing to the right) at which the informational label is to be written.

The default value of 'ILA' is 0.

'ILB' - Informational Label Box Flag - Integer

If 'ILB' is zero, the informational label will not be boxed at all. The value 1 implies that the perimeter of the box is to be drawn (in the same color as the label) after the label is drawn. The value 2 implies that the box is to be filled (in the color specified by 'LBC') before the label is drawn. The value 3 implies both of the above.

The default value of 'ILB' is 0.

'ILC' - Informational Label Color Index - Integer

If 'ILC' is less than zero, the informational label and the box, if any, around it, will be drawn in the color specified by the current text color index; if 'ILC' is greater than or equal to zero, then it specifies the desired color index for the label and the box. If a box is drawn around the label, it is made the same color as the label itself.

The default value of 'ILC' is -1.

'ILL' - Informational Label Line Width - Real

If 'ILL' has a value less than or equal to zero, line width will not be set before drawing a box around the informational label. If 'ILL' has a value greater than zero, it specifies the desired width, as a multiple of the "normal" line width.

The default value of 'ILL' is 0.

'ILP' - Informational Label Positioning Flag - Integer

The parameter 'ILP' says how the informational label is to be positioned. There are nine possible values, each of which specifies a point of the label box which is to lie on the point defined by 'ILX' and 'ILY': the value -4 implies the lower left-hand corner of the label box, -3 implies the center of the bottom of the box, -2 the lower right-hand corner of the box, -1 the center of the left edge of the box, 0 the center of the box, +1 the center of the right edge of the box, +2 the upper left-hand corner of the box, +3 the center of the top edge of the box, and +4 the upper right-hand corner of the box. Left, right, bottom, and top are defined from the viewpoint of someone reading the label right-side up.

The default value of 'ILP' is 4, so the upper right-hand corner of the box will be placed on the point ('ILX','ILY').

'ILS' - Informational Label Size - Real

The parameter 'ILS' specifies the nominal size (width) of a character in the informational label, as a fraction of the width of the viewport. This nominal size is multiplied by 'CWM'.

The default value of 'ILS' is .012.

'ILT' - Informational Label Text String - Character

The parameter 'ILT' is a string of 128 or fewer characters, specifying the text of the informational label. The following substrings will be replaced by a numeric value:

      $CIU$ - THE CONTOUR INTERVAL USED.
      $CMN$ - THE MINIMUM CONTOUR LEVEL.
      $CMX$ - THE MAXIMUM CONTOUR LEVEL.
      $DMN$ - THE MINIMUM VALUE IN THE DATA ARRAY.
      $DMX$ - THE MAXIMUM VALUE IN THE DATA ARRAY.
      $SFU$ - THE CURRENT SCALE FACTOR.
In each case except $SFU$, the given value will have been divided by the current scale factor. A "U" may be inserted just before the final "$" (as in '$CIUU$', '$CMNU$', etc.) to request the use of an unscaled value.

The value with which $CIU$ is replaced will only be correct if CONPACKT itself has chosen the contour levels; otherwise, it may be necessary for the user to set the value of 'CIU' (which see, above).

If 'ILT' is given the value ' ' (a single blank), there will be no informational label.

The default value of 'ILT' is 'CONTOUR FROM $CMN$ TO $CMX$ BY $CIU$'.

'ILW' - Informational Label White Space Width - Real

The parameter 'ILW' specifies the nominal width of white space to be left around the informational label, as a fraction of the width of the viewport. This nominal width is multiplied by 'CWM'.

The default value of 'ILW' is .005.

'ILX' - Informational Label X Coordinate - Real

The parameter 'ILX' specifies the X coordinate of the basepoint of the informational label. The given value is mapped linearly onto the viewport; 0 refers to the left edge of the viewport and 1 to the right edge of the viewport. Values less than 0 or greater than 1 may be used.

The default value of 'ILX' is .98.

'ILY' - Informational Label Y Coordinate - Real

The parameter 'ILY' specifies the Y coordinate of the basepoint of the informational label. The given value is mapped linearly onto the viewport; 0 refers to the bottom edge of the viewport and 1 to the top edge of the viewport. Values less than 0 or greater than 1 may be used.

The default value of 'ILY' is -.02.

'IWB' - Integer Workspace for Blocking - Integer

The parameter 'IWB' specifies the amount of integer workspace to be allotted for use by CTTDBF, which sets the blocking flags for triangles in a triangular mesh being projected by the 3D package TDPACK. Increasing its value may help one of the algorithms executed by CTTDBF to run more efficiently.

The default value of 'IWM' is 2500.

'IWM' - Integer Workspace for Masking - Integer

The parameter 'IWM' specifies the amount of integer workspace to be allotted for use by CTCLDM, which draws contour lines masked by an area map, in calls to the routine ARDRLN, in the package AREAS. Assume a parameter value "n"; the space used will be "2n" ("n" for the array IAI and "n" for the array IAG, in calls to ARDRLN). The value "n" must be greater than or equal to the number of group identifiers used in generating the area map.

The default value of 'IWM' is 10.

'IWU' - Integer Workspace Usage - Integer

The parameter 'IWU' is intended for retrieval only. It is zeroed by the call to CTMESH. Thereafter, as CONPACKT routines are called, the value of 'IWU' is updated to reflect the largest number of words of integer workspace needed at any one time. Therefore, by retrieving its value after an entire plot has been constructed, one may find out how large an integer workspace was actually required.

'LBC' - Label Box Color Index - Integer

If label boxes (of whatever type) are filled, the filling is done using the color index specified by 'LBC'. If 'LBC' is less than zero, the current fill area color index is used.

The default value of 'LBC' is 0, which specifies the background color.

'LBX' - Label Box X Coordinate - Real

Not to be set by the user. The value may be retrieved in one of the routines CTCHCF, CTCHHL, CTCHIL, or CTCHLL. It specifies the X coordinate (in the current user coordinate system) of the center of the box surrounding the label that has caused the routine to be called.

The default value of 'LBX' is 0.

'LBY' - Label Box Y Coordinate - Real

Not to be set by the user. The value may be retrieved in one of the routines CTCHCF, CTCHHL, CTCHIL, or CTCHLL. It specifies the Y coordinate (in the current user coordinate system) of the center of the box surrounding the label that has caused the routine to be called.

The default value of 'LBY' is 0.

'LIS' - Label Interval Specifier - Integer

When 'CLS' is given a positive value, indicating that CONPACKT is to choose contour levels at intervals of the form "bk", where "b" is a base value and "k" is an integer, and 'CIS' is given a positive value, indicating that it is the desired value of "b", then 'LIS' must be set to specify the interval between labelled contour levels.

See the descriptions of the parameters 'CLS' and 'CIS'.

As an example, one might specify 'CLS'=1, 'CIS'=1/3 and 'LIS'=3 in order to get contours at values like 1/3, 2/3, 3/3, 4/3, etc., with labels at values like 1, 2, 3, etc.

The default value of 'LIS' is 5.

'LIT' - Label Interval Table - Integer Array

See the description of the parameter 'CIT'.

'LIU' - Label Interval Used - Integer

The parameter 'LIU' is for retrieval only. When CONPACKT chooses the contour interval and decides that every "nth" one should be labelled, it sets 'LIU' to "n".

'LLA' - Line Label Angle - Real

The parameter 'LLA' specifies the angle (in degrees counterclockwise from a vector pointing to the right) at which contour line labels are to be written when ABS('LLP') is greater than or equal to 2 and 'LLO' is 0.

The default value of 'LLA' is 0.

'LLB' - Line Label Box Flag - Integer

If 'LLB' is zero, contour line labels drawn by CTLBDR will not be boxed at all. The value 1 implies that the perimeter of the box is to be drawn (in the same color as the label) after the label is drawn. The value 2 implies that the box is to be filled (in the color specified by 'LBC') before the label is drawn. The value 3 implies both of the above.

The default value of 'LLB' is 0.

'LLC' - Line Label Color Index - Integer Array

Each element of the parameter array 'LLC', if greater than or equal to zero, is the color index for labels on contour lines at the level specified by the associated element of 'CLV'. A value less than zero implies that the current text color index is to be used.

This parameter only affects line labels when ABS('LLP') = 2 or 3 and the labels are therefore drawn by CTLBDR. It does not affect line labels when ABS('LLP') = 1 and the line labels are therefore drawn by a dash package routine called by CTCLDM or CTCLDR.

The default values of the elements of 'LLC' are all -1's.

'LLL' - Line Label Line Width - Real

If 'LLL' has a value less than or equal to zero, line width will not be set before drawing boxes around contour line labels. If 'LLL' has a value greater than zero, it specifies the desired width, as a multiple of the "normal" line width.

The default value of 'LLL' is 0.

'LLO' - Line Label Orientation - Integer

The parameter 'LLO' only has effect when ABS('LLP') is greater than or equal to 2, specifying use of either the regular scheme or the penalty scheme for positioning labels on contour lines. If 'LLO' is zero, the labels are written at the angle specified by 'LLA'. If 'LLO' is non-zero, the labels are written in the local direction of the contour line.

The default value of 'LLO' is 0.

'LLP' - Line Label Positioning - Integer

The parameter 'LLP' says whether or not labels should be produced and, if so, how, as follows:

When 'LLP' is 2 or 3, the 2D smoothing, if any, implied by the value of 'T2D' is suspended during label positioning, so that fewer label positions will be considered; this is quite a bit faster and the results are nearly as good as if the smoothing were done. To force smoothing, use 'LLP' = -2 or -3.

The default value of 'LLP' is 1.

'LLS' - Line Label Size - Real

The parameter 'LLS' specifies the nominal size (width) of a character in a contour line label written by one of the schemes used when 'LLP' is 2 or 3; the value of 'LLS' is given as a fraction of the width of the viewport. This nominal size is multiplied by 'CWM'.

The value of 'LLS' does not affect contour line labels being written by a dash package, as happens when 'LLP' has the value 1. The width of the characters used in writing those labels is determined instead by the value of the internal parameter 'DPS'.

The default value of 'LLS' is .010.

'LLT' - Line Label Text String - Character

For each I from 1 to 'NCL', element I of the parameter array 'LLT' is a string of 64 or fewer characters, to be used as a label for the contour level specified by the Ith element of 'CLV'. Since the character string will be plotted using the routine PLCHHQ, in the package PLOTCHAR, it may contain colon-enclosed "function codes" to do things like create superscripts.

If the elements of this array are not supplied by the user, they will be filled in by CONPACKT itself.

'LLW' - Line Label White Space - Real

The parameter 'LLW' specifies the nominal width of white space to be left around a contour line label, as a fraction of the width of the viewport. This nominal width is multiplied by 'CWM'.

The default value of 'LLW' is .005.

'LOC' - Low Label Color Index - Integer

The parameter 'LOC' is used in determining the color index for low labels. See the description of 'HLC', above.

'LOT' - Low Label Text String - Character

The parameter 'LOT' specifies the text string to be used in labelling a low. See 'HLT', above.

'MAP' - Mapping Flag - Integer

If 'MAP' is zero, it says that the X and Y coordinates used to create the contour map are to be just the X and Y coordinates of the triangular mesh; the Z coordinates are discarded. If 'MAP' is non-zero, it says that the X, Y, and Z coordinates are to be transformed by calling the user-replaceable subroutine CTMXYZ to obtain X and Y coordinates in the user system in which drawing is done. So far, the default version of CTMXYZ provides two useful mappings:

Using any other non-zero value of 'MAP' will result in the identity mapping.

Of course, one can replace the routine CTMXYZ and build as many different mappings into it as desired, but the above mappings should be included. See the description of CTMXYZ.

The default value of 'MAP' is 0.

'NCL' - Number of Contour Levels - Integer

If CONPACKT is to pick contour levels (see the description of the parameter 'CLS') then the initial call to CTMESH causes 'NCL' to be zeroed. Subsequently, during the first call to a CONPACKT routine requiring contour levels to have been chosen, 'NCL' will be set as part of the process of choosing them. If the user elects to choose the contour levels, the first parameter which must be set to do this is 'NCL'.

The parameter 'NCL' has no meaningful default value.

'NEL' - Numeric Exponent Length - Integer

Giving 'NEL' a value less than or equal to zero says that exponents in numeric labels should be written in the shortest possible form; plus signs are omitted and the exponent magnitude is written with no leading zeroes. A value "n" which is greater than zero indicates that all exponents should be written with a sign (+ or -) and that the exponent magnitude should be padded with leading zeroes to a length of n characters.

The default value of 'NEL' is 0.

'NET' - Numeric Exponent Type - Integer

The parameter 'NET' says what characters are to be used between the mantissa of a numeric label and the exponent. The value 0 implies the use of an E, as in FORTRAN "E format", the value 1 implies the use of function codes, as expected by the utility PLOTCHAR, to generate "x 10n", where n is a superscript exponent, and the value 2 implies the use of "x10**".

The default value of 'NET' is 1.

'NEU' - Numeric Exponent Use Flag - Integer

Giving 'NEU' a value less than or equal to zero forces the use of the exponential form in all numeric labels. A positive value "n" indicates that the form without an exponent should be used as long as it requires no more than n characters; otherwise the form requiring the fewest characters should be used.

The default value of 'NEU' is 5.

'NLS' - Numeric Leftmost Significant Digit Flag - Integer

Giving 'NLS' the value zero says that the leftmost non-zero digit of a number represented by a numeric label is to be considered its first significant digit. A non-zero value says that the digit in the same digit position as the leftmost non-zero digit of the largest number (in absolute value) in the data field being contoured is to be considered the leftmost significant digit. This tends to make the numeric labels more nearly consistent with one another. Consider the following example, using three significant digits:

      USING 'NLS'=0: .500 1.00 1.50 ... 9.50 10.5 ...
      USING 'NLS'=1: .5   1.0  1.5  ... 9.5  10.5 ...
The default value of 'NLS' is 1.

'NLZ' - Numeric Leading Zero Flag - Integer

Giving 'NLZ' a non-zero value says that a zero is to placed before any numeric label which would otherwise begin with a decimal point (use "0.345", rather than ".345").

The default value of 'NLZ' is 0.

'NOF' - Numeric Omission Flags - Integer

The parameter 'NOF' says what parts of a numeric label may be omitted. The value 0 says that no part may be omitted. Add a 4 to indicate that a leading "1" or "1." which is unnecessary (as in "1 x 1013") may be omitted, a 2 to indicate that a trailing decimal point (as in "13.") may be omitted, and a 1 to indicate that trailing zeroes (as in "46.200") may be omitted.

Contour line labels generated by CONPACKT and values in the informational label which are known to have been rounded to "nice" values (like '$CIU$', '$CMN$', and '$CMX$') will have trailing zeroes trimmed in any case, no matter what the value of 'NOF' is.

The default value of 'NOF' is 6 (4 + 2).

'NSD' - Number of Significant Digits - Integer

The parameter 'NSD' specifies the maximum number of significant digits to be used in numeric labels representing contour field values. A negative value "-n" indicates that n significant digits should be used. A positive value "n" indicates that "m+n" digits should be used, where "m" is the number of digits that are the same for all values in the contour field. (For example, if the minimum value is "1123.6" and the maximum value is "1125.9", then the value of "m" is 3.)

The default value of 'NSD' is 4.

'NVS' - Number of Vertical Strips - Integer

When the parameter 'NVS' is non-zero, an extra group of edges, with group identifier 'GIS', is added to the area map by the routine CTCLAM. These edges include the boundary of the viewport and enough vertical lines to break the area occupied by the viewport up into 'NVS' vertical strips. The object of this is to break up the contour bands which are to be filled into smaller and simpler pieces; this may be necessary if the graphics device in use limits the number of points which may be used to define a polygon to be filled. The area identifier for the outside of the viewport is -1; all other area identifiers used are 0's.

The default value of 'NVS' is 1.

'ORV' - Out-of-Range Value - Real

If 'ORV' is non-zero, it specifies an out-of-range value, to be used as the value of X and Y coordinates returned by the mapping routine CTMXYZ to say that a point is out-of range (invisible) under the current mapping.

When 'MAP' = 1, a good value for 'ORV' is 1.E12, since that value is returned by EZMAP routines to signal such a point.

The default value of 'ORV' is 0.

'PAI' - Parameter Array Index - Integer

The value of 'PAI' must be set before calling CTGETC, CTGETI, CTGETR, CTSETC, CTSETI, or CTSETR to access any parameter which is an array; it indicates which element of the array is meant. For example, to set the 10th contour level to zero, use code like this:

      CALL CTSETI ('PAI - PARAMETER ARRAY INDEX',10)
      CALL CTSETR ('CLV - CONTOUR LEVEL',0.)
The default value of 'PAI' is 0.

'PC1' - Penalty Scheme Constant 1 - Real

The parameter 'PC1' is one of the constants used in the penalty scheme for positioning labels. The largest gradient allowed at the position of a label will be GRAV + 'PC1' * GRSD, where GRAV is the average gradient and GRSD is the standard deviation of the gradients.

The default value of 'PC1' is 1.

'PC2' - Penalty Scheme Constant 2 - Real

The parameter 'PC2' is one of the constants used in the penalty scheme for positioning labels. It specifies the maximum (estimated) number of contour bands allowed to cross a label.

The default value of 'PC2' is 5.

'PC3' - Penalty Scheme Constant 3 - Real

The parameter 'PC3' is one of the constants used in the penalty scheme for positioning labels. It specifies, in degrees, the maximum cumulative change in direction to be allowed along that portion of the contour line covered by a circle centered on a label and having a radius equal to half the width of the label.

The default value of 'PC3' is 60.

'PC4' - Penalty Scheme Constant 4 - Real

The parameter 'PC4' is one of the constants used in the penalty scheme for positioning labels. It specifies the "optimal distance" in the term in the penalty function which attempts to force labels to be at an optimal distance from each other.

The default value of 'PC4' is .05.

'PC5' - Penalty Scheme Constant 5 - Real

The parameter 'PC5' is one of the constants used in the penalty scheme for positioning labels. It specifies the "folding distance" in the term in the penalty function which attempts to force labels to be at an optimal distance from each other.

The default value of 'PC5' is .15.

'PC6' - Penalty Scheme Constant 6 - Real

The parameter 'PC6' is one of the constants used in the penalty scheme for positioning labels. It specifies the minimum distance to be allowed between any two labels on the same contour line, as a fraction of the width of the viewport.

The default value of 'PC6' is .30.

'PIC' - Point Interpolation Flag for Contours - Integer

The parameter 'PIC' specifies the number of points to interpolate between each pair of points defining a segment of a contour line, prior to any mapping implied by the parameter 'MAP'. It is intended that a non-zero value should normally be used only if 'MAP' is non-zero, which turns mapping on, and 'T2D' is zero, which turns the 2D smoother off; the intent is to map straight-line segments of contour lines more nearly correctly into curved-line segments on a background (one drawn by EZMAP, for example). If the 2D smoother is turned on, the additional points will be used and the smoothed curve will be constrained to pass through them; this may be useful.

A negative value of 'PIC' causes ABS('PIC') points to be interpolated, but the interpolated points are not, in general, used to draw the line segment; the object, in this case, is simply to do a finer search for changes in visibility (out-of-range state, as defined by values of 'ORV' returned by the routine CTMXYZ) along the segment.

See also the description of the parameter 'PIT', which can be used to cause the interpolation of points on segments of contour lines only in regions where the mapping causes great distortion.

The default value of 'PIC' is 0.

'PIE' - Point Interpolation Flag for Edges - Integer

The parameter 'PIE' specifies the number of points to interpolate between each pair of points defining a segment of an "edge" (the edge of the grid or the edge of an out-of-range area). It is intended that a non-zero value should normally be used only if 'MAP' is non-zero, which turns mapping on; the intent is to map straight-line segments of edge lines more nearly correctly into curved-line segments on a background (one drawn by EZMAP, for example).

A negative value of 'PIE' causes ABS('PIE') points to be interpolated, but the interpolated points are not, in general, used to draw the line segment; the object, in this case, is simply to do a finer search for changes in out-of-range state (visibility) along the segment. (The edges of out-of-range areas, however, are drawn using all such interpolated points.)

See also the description of the parameter 'PIT', which can be used to cause the interpolation of points on segments of edges only in regions where the mapping causes great distortion.

The default value of 'PIE' is 0.

'PIT' - Point Interpolation Threshold - Real

When 'MAP' is non-zero, the parameter 'PIT' may also be set non-zero to improve the accuracy with which contour lines and edge lines are mapped. As the points defining such a line are mapped (using CTMXYZ), CONPACKT looks for a jump in the mapped X coordinates of more than 'PIT' times the width of the current user-coordinate-system window or a jump in the mapped Y coordinates of more than 'PIT' times the height of the current user-coordinate-system window. When such a jump is seen, additional points are interpolated and mapped to reduce all such jumps below the threshold value. This parameter becomes particularly important when using some of the EZMAP projections that project the entire globe to the interior of a circle, in which case the points defining a segment of a contour line can map to points so far apart that the mapped contour lines cross one another.

The use of the internal parameter 'PIT' does not entirely replace the use of 'PIC' and 'PIE'; indeed, there are situations in which both can be used to good advantage, particularly when negative values of 'PIC' and 'PIE' are used to improve the resolution of the search for changes in out-of-range state along the line segments.

The default value of 'PIT' is 0. It's probably not a good idea to use values less than about .001 or greater than about .1.

'PW1' - Penalty Scheme Weight 1 - Real

The parameter 'PW1' specifies the weight for the gradient term in the penalty function.

The default value of 'PW1' is 2.

'PW2' - Penalty Scheme Weight 2 - Real

The parameter 'PW2' specifies the weight for the number-of-contours term in the penalty function.

The default value of 'PW2' is 0.

'PW3' - Penalty Scheme Weight 3 - Real

The parameter 'PW3' specifies the weight for the change-in-direction term in the penalty function.

The default value of 'PW3' is 1.

'PW4' - Penalty Scheme Weight 4 - Real

The parameter 'PW4' specifies the weight for the optimum-distance term in the penalty function.

The default value of 'PW4' is 1.

'RC1' - Regular Scheme Constant 1 - Real

The parameter 'RC1' specifies the desired distance from the beginning of a contour line to the first label on that line when they are positioned using the "regular" scheme. The nth label on each labelled contour line will be at a distance 'RC1' + 'RC2' x (n-1) + 'RC3' x Rn units (in the fractional coordinate system) from the beginning of the line, where "Rn" is a random number between -1 and 1.

The default value of 'RC1' is .25.

'RC2' - Regular Scheme Constant 2 - Real

The parameter 'RC2' specifies the desired nominal distance between labels when they are positioned using the "regular" scheme. See the description of 'RC1', above.

The default value of 'RC2' is .25.

'RC3' - Regular Scheme Constant 3 - Real

The parameter 'RC3' specifies the desired maximum variation in the distance between labels when they are positioned using the regular scheme. See the description of 'RC1', above.

The default value of 'RC3' is .05.

'RWC' - Real Workspace for Contours - Integer

The parameter 'RWC' specifies the amount of real workspace to be allotted to hold X coordinates of points defining contour lines. Assume a parameter value "n". If no 2D smoothing is requested, the total space used will be "2n" ("n" for X coordinates and another "n" for Y coordinates). If 2D smoothing is requested, the total space used will be "7n" ("n" for X coordinates, "n" for Y coordinates, and "5n" for scratch arrays).

The same contour lines are produced when using a small value of 'RWC' as would be produced when using a larger value. However, there are times when a larger value should be used; relevant facts are as follows:

The default value of 'RWC' is 1000.

'RWG' - Real Workspace for Gradients - Integer

The parameter 'RWG' specifies the amount of real workspace to be allotted to hold gradients which are to be computed and used in positioning labels using the penalty scheme. Up to a point, using a larger value provides for a more accurate representation of the gradient field.

The default value of 'RWG' is 1000.

'RWM' - Real Workspace for Masking - Integer

The parameter 'RWM' specifies the amount of real workspace to be allotted for use by CTCLDM, which draws contour lines masked by an area map, in calls to the routine ARDRLN, in the package AREAS. Assume a parameter value "n"; the space used will be "2n" ("n" for the X-coordinate array XCS and "n" for the Y-coordinate array YCS, in calls to ARDRLN). Any value of "n" greater than or equal to 2 will work; smaller values will cause the generation of more calls to the user routine RTPL (one of the arguments of CTCLDM).

The default value of 'RWM' is 100.

'RWU' - Real Workspace Usage - Integer

The parameter 'RWU' is intended for retrieval only. It is zeroed by the call to CTMESH. Thereafter, as CONPACKT routines are called, the value of 'RWU' is updated to reflect the largest number of words of real workspace needed at any one time. Therefore, by retrieving its value after an entire plot has been constructed, one may find out how large a real workspace was actually required.

'SET' - Do-SET-Call Flag - Integer

Giving 'SET' the value 0 says that no SET call is to be done by CONPACKT; the value 1 says that it is to be done. In the latter case, the call is done by CTMESH.

Arguments 5-8 of a SET call done by the user must be consistent with the ranges of the X and Y coordinates being used by CONPACKT, as specified by the X, Y, and Z coordinates of the points of the triangular mesh, the value of the parameter 'MAP', and, if 'MAP' is non-zero, the code of the routine CTMXYZ.

The default value of 'SET' is 1.

'SFS' - Scale Factor Selector - Real

The scale factor is that value (usually, but not necessarily, a power of 10) by which the actual values of contour field values are to be divided to get the value of a numeric label. If 'SFS' is given a value greater than zero, that value is the scale factor to be used. If 'SFS' is given a value less than or equal to zero, it is truncated to form an integer directing CONPACKT to select a scale factor, as follows:

The default value of 'SFS' is 1.

'SFU' - Scale Factor Used - Real

The parameter 'SFU' is intended for retrieval only; it gives the value of the scale factor selected for use by CONPACKT.

'SSL' - Smoothed Segment Length - Real

The parameter 'SSL' specifies the distance between points used to draw the curves generated by 2D smoothing; it is expressed as a fraction of the width of the window in the coordinate system in which the smoothing is being done.

The default value of 'SSL' is .01.

'TBA' - Triangle Blocking Mask - AND - Integer

Associated with each triangle of a triangular mesh is a "blocking flag", the low-order twelve bits of which may be used to "block" the triangle (in effect, remove it from the mesh). Each of the twelve bits may be associated with a particular condition and each of those conditions can be tested individually. If ITBF is the twelve-bit value of a particular triangle's blocking flag, ITBA is the value of 'TBA', and ITBX is the value of 'TBX', then the triangle will be considered blocked if and only if the quantity IAND(IXOR(ITBF,ITBX),ITBA) is non-zero (where IAND and IXOR are functions that perform the logical operations AND and EXCLUSIVE-OR, respectively, of two twelve-bit operands). (Note, however, that the routines CTMESH, CTTDCA, and CTTDBF use the function IAND(IAND(IXOR(ITBF,ITBX),ITBA),1) instead, so as to examine only the user-set blocking bit.)

Using a "1" for one of the twelve bits of 'TBX' causes the corresponding bit of a triangle blocking flag to be toggled, so that its meaning is reversed. Using a "1" for one of the twelve bits of 'TBA' causes the corresponding bit of a triangle blocking flag to be examined, so that its value is significant in determining blocking of the triangle.

By convention, the rightmost bit of a triangle blocking flag is reserved for direct blocking of triangles by the user of CONPACKT. See the routines CTTDBF and CTTDBM for a description of a scheme that uses a total of six other bits to effect blocking of triangles that are hidden, edge-on, and/or seen from the wrong side by one of the two eyes in the case where CONPACKT is being used in conjunction with TDPACK to draw a perspective view of a triangular mesh.

The default value of 'TBX' is 0; none of the bits of a triangle blocking flag are toggled.

The default value of 'TBA' is 1; only the lowest bit of a triangle blocking flag is significant.

'TBX' - Triangle Blocking Mask - XOR - Integer

See the description of 'TBA', above.

The default value of 'TBX' is 0; none of the bits of a triangle blocking flag are toggled.

'T2D' - Tension on 2-Dimensional Splines - Real

A non-zero value of 'T2D' says that 2D smoothing (using cubic splines under tension) should be done; the absolute value of 'T2D' is the desired tension. If 'T2D' is negative, smoothing will be done before the mapping, if any, requested by the flag 'MAP'; if 'T2D' is positive, smoothing will be done after the mapping.

The default value of 'T2D' is 0.

'VPB' - Viewport Bottom - Real

The parameter 'VPB' is only used when 'SET' is non-zero, saying that CONPACKT should do the call to SET; it specifies the position of the bottom edge of the area in which the viewport is to be placed, expressed as a fraction between 0 (the bottom edge of the plotter frame) and 1 (the top edge of the plotter frame). See also the description of 'VPS'.

The default value of 'VPB' is .05.

'VPL' - Viewport Left - Real

The parameter 'VPL' is only used when 'SET' is non-zero, saying that CONPACKT should do the call to SET; it specifies the position of the left edge of the area in which the viewport is to be placed, expressed as a fraction between 0 (the left edge of the plotter frame) and 1 (the right edge of the plotter frame). See also the description of 'VPS'.

The default value of 'VPL' is .05.

'VPR' - Viewport Right - Real

The parameter 'VPR' is only used when 'SET' is non-zero, saying that CONPACKT should do the call to SET; it specifies the position of the right edge of the area in which the viewport is to be placed, expressed as a fraction between 0 (the left edge of the plotter frame) and 1 (the right edge of the plotter frame). See also the description of 'VPS'.

The default value of 'VPR' is .95.

'VPS' - Viewport Shape - Real

The parameter 'VPS' is only used when 'SET' is non-zero, saying that CONPACKT should do the call to SET; it specifies the desired viewport shape, as follows:

The viewport, whatever its final shape, is centered in, and made as large as possible in, the area specified by the parameters 'VPB', 'VPL', 'VPR', and 'VPT'.

The default value of 'VPS' is .25.

'VPT' - Viewport Top - Real

The parameter 'VPT' is only used when 'SET' is non-zero, saying that CONPACKT should do the call to SET; it specifies the position of the top edge of the area in which the viewport is to be placed, expressed as a fraction between 0 (the bottom edge of the plotter frame) and 1 (the top edge of the plotter frame). See also the description of 'VPS'.

The default value of 'VPT' is .95.

'WDB' - Window Bottom - Real

When CONPACKT does the call to 'SET', the parameter 'WDB' is used to determine argument number 7, the user Y coordinate at the bottom of the window. If 'WDB' is not equal to 'WDT', 'WDB' is used. If 'WDB' is equal to 'WDT', then CTMESH computes a value to use by examining all non-blocked triangles of the mesh.

The default value of 'WDB' is 0.

'WDL' - Window Left - Real

When CONPACKT does the call to 'SET', the parameter 'WDL' is used to determine argument number 5, the user X coordinate at the left edge of the window. If 'WDL' is not equal to 'WDR', 'WDL' is used. If 'WDL' is equal to 'WDR', then CTMESH computes a value to use by examining all non-blocked triangles of the mesh.

The default value of 'WDL' is 0.

'WDR' - Window Right - Real

When CONPACKT does the call to 'SET', the parameter 'WDR' is used to determine argument number 6, the user X coordinate at the right edge of the window. If 'WDR' is not equal to 'WDL', 'WDR' is used. If 'WDR' is equal to 'WDL', then CTMESH computes a value to use by examining all non-blocked triangles of the mesh.

The default value of 'WDR' is 0.

'WDT' - Window Top - Real

When CONPACKT does the call to 'SET', the parameter 'WDB' is used to determine argument number 8, the user Y coordinate at the top of the window. If 'WDT' is not equal to 'WDB', 'WDT' is used. If 'WDT' is equal to 'WDB', then CTMESH computes a value to use by examining all non-blocked triangles of the mesh.

The default value of 'WDT' is 0.

'WSO' - Workspace Overflow Flag - Integer

The parameter 'WSO' says what to do when a real or integer workspace overflow occurs, as follows:

When execution continues, the resulting plot will be incomplete. The values of 'IWU' and 'RWU' may be retrieved to find out how much workspace would have been used if the call on which the workspace overflow occurred had succeeded; note, however, that if these amounts are provided on a subsequent run, one is still not assured that the workspace overflow will be averted.

The default value of 'WSO' is 1.

'XMN' - X Minimum Value - Real

The minimum value of X in the mesh, as found by CTMESH. For output only.

'XMX' - X Maximum Value - Real

The maximum value of X in the mesh, as found by CTMESH. For output only.

'YMN' - Y Minimum Value - Real

The minimum value of Y in the mesh, as found by CTMESH. For output only.

'YMX' - Y Maximum Value - Real

The maximum value of Y in the mesh, as found by CTMESH. For output only.

'ZMN' - Z Minimum Value - Real

The minimum value of Z in the mesh, as found by CTMESH. For output only.

'ZMX' - Z Maximum Value - Real

The maximum value of Z in the mesh, as found by CTMESH. For output only.

ERROR HANDLING

When a CONPACKT routine detects an error condition, it calls the routine SETER, which is the principal routine in the error-handling package for NCAR Graphics. (There is a programmer document describing SETER and associated routines; see the SETER document for complete information about error handling in NCAR Graphics.)

By default, SETER prints a line and STOPs. The line printed will look something like this:

    ERROR    3 IN CTGRWS - REAL WORKSPACE OVERFLOW
The error number ("3", in the example) may be of use to a consultant (to determine exactly where the error occurred), but is not otherwise meaningful. The actual error message consists of the name of the routine in which the error occurred ("CTGRWS", in the example), a blank, a minus sign, another blank, and, lastly, a short description of the error.

All errors are "recoverable" in the sense that, if the user program puts SETER into "recovery mode", control will be returned to the caller of the CONPACKT routine in which the error occurred. In some cases, it is then possible to take remedial action to get around whatever problem has occurred; in any case, the error flag can be cleared and execution of the user's program can continue.

When SETER is in recovery mode (and, occasionally, even when it is not), error messages may have a somewhat more complicated form, like this:

    CTCLDR/CTGRWS - REAL WORKSPACE OVERFLOW
What this particular error message says is that CTCLDR called CTGRWS, which detected an error condition (real workspace overflow) and called SETER. Upon getting control back from CTGRWS, CTCLDR detected the fact that CTGRWS had logged an error. It augmented the error message by prepending its own name, followed by a slash, and then passed control back to the user. Of course, there can be more than two such levels of routine calls indicated in the error message: in a few cases, seven or eight routine names may be listed, each separated from the next by a slash.

The various error conditions in CONPACKT are described in the list below. Each bulleted item includes an error message and a thumb-nail description of the error. The items in the list are arranged in alphabetical order. If you get an error message with one or more prefixed subroutine names, as described above, omit them and look up the result in this list. Note that, since CONPACKT routines sometimes call other routines, elsewhere in NCAR Graphics, that can detect error conditions and call SETER, the error message you get by calling a CONPACKT routine may not be listed here, but in the programmer document for some other package.

This probably indicates an error in the implementation of the package. See the CONPACKT specialist.

The contour-level list has more than one entry for a given level (which is okay) but either the values of 'AIA' for that level or the values of 'AIB' for that level are inconsistent.

One of the points P and Q, which determine how the cell array is to be mapped onto the plotter frame, is outside the limits of the plotter frame.

Either the specified first dimension of the FORTRAN array in which the cell array is stored is less than or equal to zero or one of the specified dimensions of the cell array itself is less than or equal to zero or the specified first dimension of the cell array is larger than the specified first dimension of the FORTRAN array in which it is stored.

One of the routines CTTMRG, CTTMTL, or CTTMTX has been given an edge array (IEDG) which is too small. Increase its size.

An attempt to get the current clipping state has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get the current normalization transformation number has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get a color index has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get a line width scale factor has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get the current normalization transformation has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get a color index has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt to get a color index has resulted in an error. This probably indicates that GKS is in the wrong state.

An attempt has been made to get an element of the parameter array named 'xxx' and the current value of 'PAI' (the "parameter array index") is inappropriate for that parameter array.

A call to CTMESH has been omitted.

The parameter 'WSO' has a value indicating that integer workspace overflow should be treated as a fatal error, and such an overflow has occurred.

An attempt has been made to set the number of contour levels to an illegal value.

CTMVIW or CTMVIW has been called to move the contents of an old workspace array to a new one, and the new one is too small.

The given parameter name ('xxx') is not one of the legal parameter names.

The parameter name ('x') is less than three characters long.

One of the routines CTTMRG, CTTMTL, or CTTMTX has been given a point array (RPNT) which is too small. Increase its size.

The parameter 'WSO' has a value indicating that real workspace overflow should be treated as a fatal error, and such an overflow has occurred.

An attempt has been made to set an element of the parameter array named 'xxx' and the current value of 'PAI' (the "parameter array index") is inappropriate for that parameter array.

Indicates that the parameter 'CLS' has been given a negative value whose absolute value is too large, requesting more than 256 contour levels.

One of the routines CTTMRG, CTTMTL, or CTTMTX has been given a triangle array (ITRI) which is too small. Increase its size.

This error message indicates that, at the time the routine was called, there was an unrecovered outstanding error. In this case, it cannot continue; it forces the error message for the outstanding error to be printed and then substitutes this one for it.

EXAMPLES

Twenty-four examples are available for CONPACKT. On a Unix system on which NCAR Graphics has been installed, one may obtain the code for an example and run it by executing the command

      ncargex example_name
where "example_name" is one of the following:

      ctex01 ctterr ctex02 ctllg1 ctllg2 ctllg3 ctpopg ctorca
      ctiscp ctisc2 ctwng1 ctwng2 ctgeo1 ctgeo2 ctgeo3 ctgc23
      ctfite ctgaus ctswth ctcbay ctnccl cttd01 cttd02

      c_ctllg3
To obtain the code for an example without running it, one uses the command

      ncargex -n example_name
After the code has been examined and, perhaps, modified, it can be compiled and executed using the commands

      ncargf77 *.f
      a.out
The metafile created by executing an example can be viewed using the command

      ctrans metafile_name
(It may be necessary to set some environment variables and/or to set other options on the "ctrans" command line.)

Most of the example code is heavily commented to explain what is being done and it is heavily parameterized. For example, one can easily specify the nature of the workstation being used (NCGM, X Windows, PostScript, or PDF in portrait or landscape mode). In many cases, one can select which of the plots an example can draw will actually be drawn and what the resolution of the triangular mesh used in the example will be. In the case of 3D plots, one can easily change the vantage point from which the mesh is viewed, its distance from the eye, whether stereo views or a single view will be drawn, and so on.

The Example "ctex01"

The example "ctex01" produces three frames illustrating the use of CONPACKT to draw contours on a simple triangular mesh derived from a rectangular grid in the plane and it shows, in great detail, how the mesh was constructed. If you want to learn how to do that, this is the best example to study; if not, you may find one of the other examples somewhat easier to follow, since, in general, those examples use one of the two canned routines in CONPACKT to construct a triangular mesh.

The Example "ctterr"

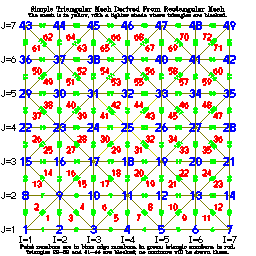
The example "ctterr" is just like "ctex01", except that it has been adapted to process a rectangular array of user data representing a vertical slice through an ocean basin. The rectangular grid follows the terrain of the ocean bottom. These data came to us from Jim Hawkins, who writes:

"If you want to describe the context of our work ... we are studying the interaction of acoustic waves with internal gravity waves. The internal gravity waves are modeled with ocean/atmosphere models. (The one that generated the data I sent you was generated by a 2D model designed by Professor Kevin Lamb of the University of Waterloo, Waterloo, Ontario, and we have used one written by Dr. Piotr Smolarkiewicz of NCAR, Boulder). The data are on what we call 'sigma' coordinates or terrain following coordinates ... "

The Example "ctex02"

The example "ctex02" produces ten frames illustrating the use of CONPACKT to draw contours on a planar triangular mesh that is created in such a way as to fill an area having an irregular boundary, including some holes. The area chosen is, by default, the conterminous 48 states of the US, as seen on an orthographic projection of the globe, but the program could easily be modified to work for an area defined differently. Click on the image below for more information:

The Example "ctllg1"

The example "ctllg1" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh created from a simple rectangular grid mapped onto the globe. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctllg2"

The example "ctllg2" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh created from a simple rectangular grid mapped onto the globe. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctllg3"

The example "ctllg3" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh created from a simple rectangular grid mapped onto the globe. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctpopg"

The example "ctpopg" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a POP grid on the globe. These data came to us from Frank Bryan, who describes them as "data from the low-resolution version of the CCSM3 ocean component model".

Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctorca"

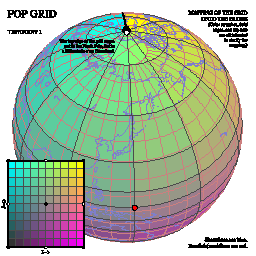
The example "ctorca" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from an ORCA grid on the globe. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctiscp"

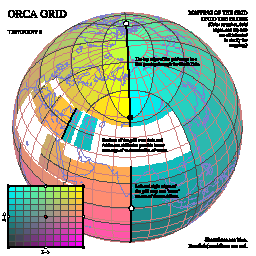
The example "ctiscp" produces twenty frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from an ISCCP grid on the globe. Five different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctisc2"

The example "ctisc2" produces twenty frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from an ISCCP grid on the globe. Five different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctwng1"

The example "ctwng1" produces twenty frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a SEAM grid (obtained from Houjun Wang) on the globe. Five different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctwng2"

The example "ctwng2" produces twenty frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a SEAM grid (obtained from Houjun Wang) on the globe. Five different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctgeo1"

The example "ctgeo1" produces sixteen frames illustrating the use of CONPACKT to draw contours on a triangular geodesic mesh that is created by subdividing the triangular faces of an icosahedron into smaller triangles and projecting them onto the surface of the globe. Four different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctgeo2"

The example "ctgeo2" produces sixteen frames illustrating the use of CONPACKT to draw contours on a triangular geodesic mesh that is created by subdividing the triangular faces of an icosahedron into smaller triangles and projecting them onto the surface of the globe. The method used is a bit different from that used in "ctgeo1". Four different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctgeo3"

The example "ctgeo3" produces sixteen frames illustrating the use of CONPACKT to draw contours on a triangular geodesic mesh obtained by downloading data from a Web site at Colorado State University:

http://kiwi.atmos.colostate.edu/BUGS/geodesic/interpolate.html

The scientists who provided the data included David Randall, Ross Heikes, and/or Todd Ringler. This mesh was obviously created by subdividing the triangles of an icosahedron into smaller triangles and projecting them onto the surface of the globe, but some mathematical technique was then used to alter the mesh slightly so as to make all of its triangles have more nearly the same size. (See the example "ctgc23" for plots comparing the resulting mesh with that produced by "ctgeo2".) Four different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctgc23"

The example "ctgc23" produces four frames comparing the geodesic grids of examples "ctgeo2" and "ctgeo3". Frame 1 shows both grids in orthographic projection on the globe, frame 2 shows both grids in a close-up view of the same projection, frame 3 shows a histogram of the areas of the triangles of the grid of example "ctgeo2", and frame 4 shows a histogram of the areas of the triangles of the grid of example "ctgeo3", showing clearly how much more nearly equal in size they are.

The Example "ctfite"

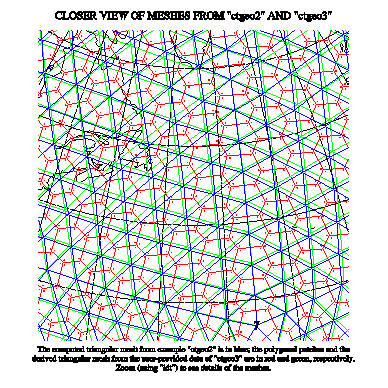
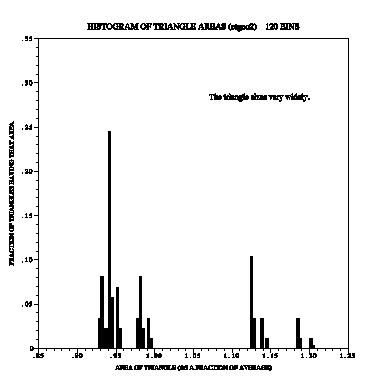
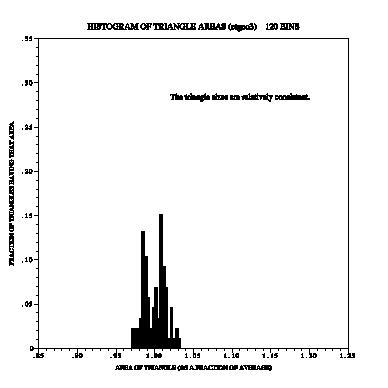
The example "ctfite" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a POP grid on the globe. This particular grid came from Marika Holland; the data are ice thicknesses in polar oceans. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctgaus"

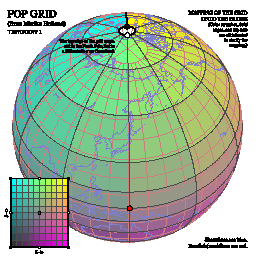
The example "ctgaus" produces twenty-four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a Gaussian grid on the globe. This particular grid came from Paul Swarztrauber; dummy data are used on it. Six different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctswth"

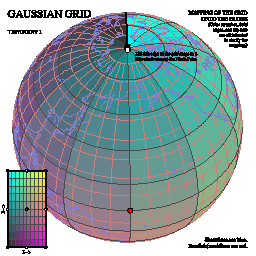
The example "ctswth" produces sixteen frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is created from a rectangular mesh that came from Simona Bordoni. The data seem to be wind speeds along a satellite track. Four different types of plots are shown for each of four different views of the globe. Click on the image below for more information:

The Example "ctcbay"

The example "ctcbay" produces four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is used to model the Chesapeake Bay and came to us from Tom Gross. The data are temperatures and came from the Chesapeake Community Model Program Quoddy model:

http://ccmp.chesapeake.org

using the NOAA/NOS standardized hydrodynamic model NetCDF format:

https://sourceforge.net/projects/oceanmodelfiles

Click on the image below for more information:

The Example "ctnccl"

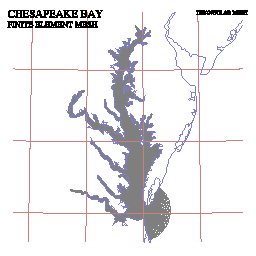
The example "ctnccl" produces four frames illustrating the use of CONPACKT to draw contours on a triangular mesh that is used to model the North Carolina coastal waters and came to us from Brett Estrade. The data are ocean depths and came from the Naval Research Laboratory at the Stennis Space Center. For more information about the grid, see the article "Application of a Shelf-Scale Model to Wave-Induced Circulation: Rip Currents" (Mark Cobb and Cheryl Ann Blain, Ocean Dynamics and Prediction Branch).

Click on the image below for more information:

The Example "cttd01"

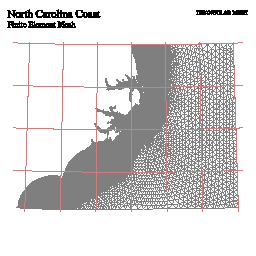
The example "cttd01" produces three frames illustrating the use of CONPACKT with the 3D package TDPACK to draw perspective views of a triangular mesh that forms a dumbbell-shaped object in 3-space. (On each frame, the two views form a crossed-eye stereo pair.) Some parts of the object are hidden behind other parts of it. The routines CTTDBF and CTTDBM are used to block triangles that are hidden by other triangles, that are seen from the wrong side, or that are too nearly edge-on to the line of sight.

Click on any of the four images to see a larger image (particularly if you want to try seeing the stereo effect).

The Example "cttd02"

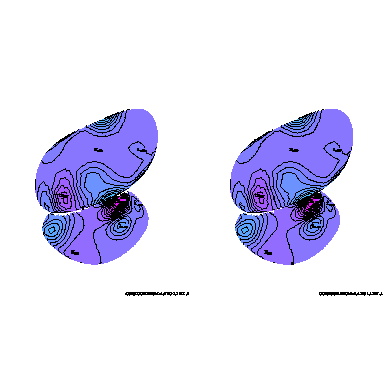
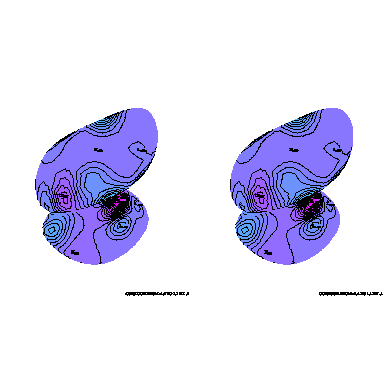
The example "cttd02" is just like "cttd01", except that the object is not completely closed, so that portions of its "interior" are visible. The routines CTTDBF and CTTDBM are used in such a way that the rectangular grid may be seen on the interior, but not the contours. For frame 3, CTTDCA is called in such a way as to make the interior yellow and the background green.

Again, click on any of the three images to see a larger image (particularly if you want to try seeing the stereo effect).

The Example "c_ctllg3"

The example "c_ctllg3" produces a single frame illustrating the use of CONPACKT to draw contours on a triangular mesh created from a simple rectangular grid mapped onto the globe. The rectangular grid is like that used in the example "ctllg3", but the code for this example is in C, rather than in FORTRAN. Click on the image below for an enlarged one: